Adsorption characteristics for the removal of a toxic dye, tartrazine from aqueous solutions by a low cost agricultural by-product

2.1 Adsorbent

Saw dust was procured from the local timber factory from Allahabad, India. Saw dust was washed several times with normal tap water followed by distilled water to remove adhered dust particles. The cleaned material was kept in an oven for 24 h at 110 °C. The dried mass was then crushed and sieved. The fraction of the particles between 0.425 and 0.6 mm (geometric mean size: 0.5 mm) was selected and used in the adsorption experiments as such without any further modification.

2.2 Adsorbate

An anionic dye, 4, 5-dihyro-5-oxo-1-(4-sulfophenyl)-4-[(4-sulfophenyl) azo]-1H pyrazole-3- carboxylic acid trisodium salt also known as Tartrazine (Acid Yellow 23, FD & C Yellow 5 , E102) with molecular formula C₁₆H₉N₄Na₃O₉S₂ and molecular weight 534.4 shown in Fig. 1 was obtained from Alfa Aesar, Great Britain with CAS No- 1934-21-0 and CI No-19140. Stock solution of the dye was made by dissolving its required amount in double distilled water. The stock solution was further diluted with distilled water to obtain the standard solutions of 1 mg/L, 5 mg/L, 10 mg/L and 15 mg/L.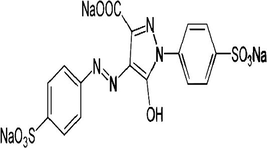

Close

Figure 1

Chemical structure of Tartrazine or Acid Yellow 23.

Export to PPT

2.3 Characterization of adsorbent

Infrared spectra of the adsorbents were obtained using a Fourier transform infrared spectrometer (ABB Model FTLA-2000, Canada). For the FT-IR study, finely ground adsorbent has been intimately mixed with KBr (Merck) in a ratio of 1:100 in order to prepare a translucent pellet. From these FT-IR spectra the presence of functional groups on the adsorbent were confirmed.

The surface morphology of the adsorbents was investigated by scanning electron microscope (Jeol JSM 6390LV). The SEM micrograph of saw dust at bar length equivalent to 200 μm with 400 magnifications, at a working voltage of 30 kV was reported.

2.4 Batch adsorption studies

In the present study batch adsorption experiments were carried out to achieve the optimum operating conditions for the removal of the selected dye. 50 mL solution of known initial concentration was taken in 250 mL Erlenmeyer flasks and a known amount of saw dust, the adsorbent was added to the solutions. The mixture was shaken mechanically at a constant speed of 180 rpm using a water bath shaker (Mac-Macro Scientific Works, Pvt Ltd, New Delhi, India). The samples were withdrawn after predetermined time viz. equilibrium time and thereafter the adsorbent was separated from the solutions by centrifugation using a centrifuge (Remi, Model-R-8CBL, India). The residual concentration of the dye in the supernatant was estimated spectrophotometrically with a double beam spectrophotometer (Model-2203 Systronics, Ahmedabad, India) at 426 nm.

2.5 Determination of pH_zpc

For the determination of pH_zpc of the adsorbent, 50 mL of 0.01 M NaCl solutions were taken in different Erlenmeyer flasks of 250 mL and 0.5 g of adsorbent was introduced in each of them. Now pH values of these solutions were adjusted in 2 to 12 range by 0.1 M HCl/NaOH solutions. These flasks were kept for 48 h and the final pH of the solutions was measured. Graphs were plotted between pH_final versus pH_initial. The point of intersection of the curve of pH_final versus pH_initial was recorded as pH_zpc of the saw dust.

2.6 Adsorption kinetics and equilibrium studies

Adsorption kinetics investigations were carried out by agitating 50 mL of dye solution of known initial concentration with 5 g/L of adsorbent at a temperature of 318 K, at a pH of 3.0 ± 0.1 and at 180 rpm for different time intervals. The amount of dye adsorbed onto the adsorbent at equilibrium, q_e (mg/g), was calculated by the following expression:

Similarly, the adsorption isotherm experiments were conducted by contacting 5 g/L of saw dust with 50 mL of dye solutions of various initial concentrations (1 mg/L, 5 mg/L, 10 mg/L, and15 mg/L). The samples were agitated on a water bath at 180 rpm at 298 K, 308 K, and 318 K for 70 min.

Blank experiments were also conducted by using dye solutions without adsorbent to ensure that no dye was adsorbed onto the containers and with adsorbent and water only to check that no leaching occurred, which would rather interfere with the measurement of dye concentrations on a spectrophotometer. All adsorption experiments were performed in triplicate and the mean values were used in data analysis.

3.1 Characterization

Fig. 2 shows the SEM micrograph of saw dust (400 X). It is clear from this image that the surface is rough and highly corrugated. Saw dust is a heterogeneous material consisting of particles of irregular shapes having considerable layers with pores of varying size and provides a fair possibility for the dye to be adsorbed.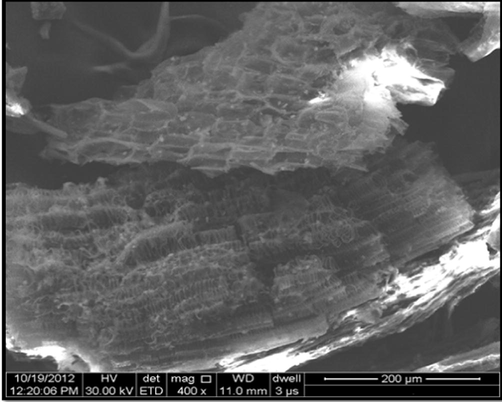

Close

Figure 2

SEM micrograph of saw dust (400 X).

Export to PPT

Sawdust is basically composed of cellulose as well as lignin, and all these components contribute as active sites for the adsorption of dye molecules. Fig. 3 displays the FT-IR spectra of sawdust in its natural form and dye loaded form. The spectra exhibited several peaks representing that saw dust is composed of various functional groups which possibly help in binding of the dye molecules. The appearance of a peak in natural saw dust at 3497 cm⁻¹ represents –OH stretching (Samiey and Ashoori, 2012). The peak at 2852 cm⁻¹ corresponds to the presence of –CH₂ stretching of aliphatic groups. The peaks at 1637 cm⁻¹ and 1321 cm⁻¹ indicate the presence of C⚌C stretching of phenol group and C–N stretching of the amine group respectively. Whereas peaks appearing at 1119 cm⁻¹ and 1032 cm⁻¹ in the spectrum contribute to C–O stretching of the phenolic group and a strong C–O bond due to ether group of cellulose, respectively (Ahmad et al., 2009). The spectra of the dye loaded saw dust showed similar characteristics as the saw dust in natural form except for slight changes. The FT-IR spectrum of the dye loaded adsorbent indicates that the peaks are slightly shifted from their position and the intensity gets altered. These results indicated the involvement of some functional group in the adsorption of dye ions on the surface of the saw dust through weak electrostatic interaction or Van der Waals forces. Therefore, there is no possibility of chemical bonding in this process. Thus the FT-IR of the surface moieties remained unchanged.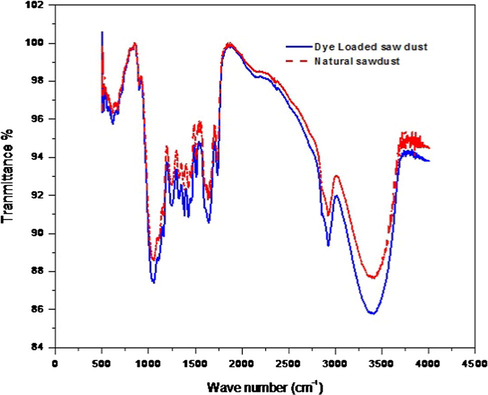

Close

Figure 3

Comparative FT-IR spectra of saw dust before and after adsorption of dye.

Export to PPT

3.2 Effect of pH

The effect of pH on the removal of tartrazine has been investigated. Solution pH plays an important role in controlling the surface charge of the adsorbent, the degree of ionization of the adsorbate in the solution as well as dissociation of various functional groups on the active sites of the adsorbent (Wawrzkiewicz and Hubicki, 2009). In most cases, pH is termed as the ‘master variable’. The adsorption experiment of anionic dye tartrazine was performed in 2–12 pH range at 318 K for 70 min. The equilibrium sorption capacity was lowest at pH 12 (0.163 mg/g) and maximum adsorption of the dye was achieved at pH 3 (0.19 mg/g). This can be explained as in the aqueous solutions, the anionic dye exists in dissociated form as anionic dye ions:

3.3 Zero point charge of adsorbent

The influence of pH on the dye removal can be illustrated on the basis of isoelectric point of the adsorbent surface. Solution pH is a significant parameter which affects the dye adsorption process. It also alters the surface charge of the adsorbent, the ionization extent of different pollutants, as well as the structure of the dye molecules (Ai et al., 2011). The presence of various ligands such as carboxyl, phosphate and amino group on lignin and cellulose based materials in the ionic state contributes to the reaction with dye ions. At pH_zpc, the acidic and basic functional groups no longer contribute to the pH of the solutions. The zero point charge, pH_zpc, of saw dust was found to be 6.32. At a pH of the solution below pH_zpc the surface of the sorbent is positively charged and can attract anions from the solution. When the solution pH is greater than pH_zpc the surface of saw dust is negatively charged and attracted cations. In the present case, a lower pH is favorable for dye removal as at low pH, the number of positively charged sites is increased which favor the adsorption of negatively charged dye ions because of the electrostatic force of attraction.

3.4 Effect of initial dye concentration and contact time

A given amount of adsorbent has the capacity to adsorb only a fixed amount of adsorbate species. Thus initial concentration of the adsorbate solution is very important. Fig. 4 shows the graph plotted between the amount of dye adsorbed (q_t) versus time, t, at different initial dye concentrations. The time variation plot indicates that the removal of dye is rapid in initial stages but when it approaches equilibrium, it slows down gradually. This may be due to the availability of vacant surface sites during the preliminary stage of adsorption, and after a certain time period the vacant sites get occupied by dye molecules which lead to create a repulsive force between the adsorbate on the adsorbent surface and in bulk phase. The attainment of equilibrium takes place after agitating the solutions containing the adsorbent up to 70 min and once equilibrium was attained, the percentage of adsorption of dye did not show any appreciable change with time. This suggests that after equilibrium is attained, further treatment does not provide more removal. In batch adsorption, the rate of removal of the adsorbate from aqueous solutions is controlled mainly by the transport of dye molecules from the surrounding sites to the interior sites of the adsorbent particles (Ahmad et al., 2009). From the corresponding plot it can be observed that the amount of dye adsorbed (mg/g) varies with varying initial dye concentration and increases with increase in initial dye concentration and after equilibrium, it becomes constant due to the driving force offered by the increased solute concentration which is sufficient enough to overcome the resistance to mass transfer between the solid and liquid phases. Hence the adsorption will enhance with higher initial concentration. When the initial dye concentration increased from 1 mg/L to 15 mg/L at 318 K and pH 3.0 ± 0.1, the sorption capacity increased from 0.186 to 1.44 mg/g while the removal percentage of dye decreased from 97% to 71%. At a higher concentration range the fractional removal is always higher whereas for low concentration ranges the percentage removal of the dye is higher (Sharma and Uma, 2010).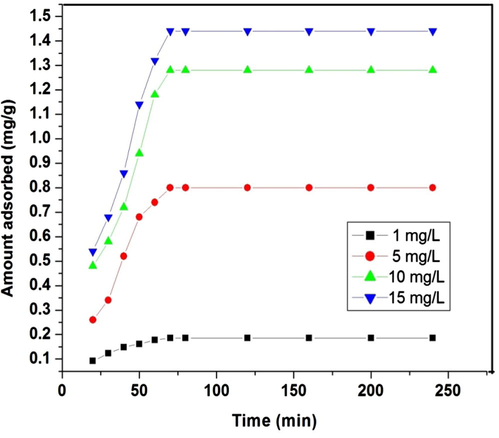

Close

Figure 4

Effect of initial dye concentration on adsorption of tartrazine. Experimental conditions: adsorbent dosage 5 g/L, contact time 240 min, pH 3.

Export to PPT

3.5 Adsorption kinetics

3.5.2

3.5.2 Pseudo-second order kinetics equation

The kinetics of the adsorption process may also be described in pseudo-second order rate equation (Ho and McKay, 1998). The linearized form of t equation is expressed as:

(5)

$$\frac{t}{q_t}=\frac{1}{k_2{q}_e^2}+\frac{t}{q_e}$$

(6)

$$h=k_2{q}_e^2$$ where the equilibrium adsorption capacity (q_e) and the second order constant k₂ (g/mg min) can be determined experimentally from the slope and intercept of plot t/q_t versus t (Fig. 5). k₂ and q_e determined from the model are presented in Table 2 along with corresponding correlation coefficients. The calculated and experimental values of q_e are presented in Table 2 and it is observed that the rate constants of pseudo second order kinetics model increased from 9.0 × 10⁻⁴ g/mg min to 2.7 × 10⁻³ g/mg min with an increase in temperature indicating that the sorption system process reached equilibrium faster at high temperature. This may be due to an increase in the probability of collision between active surface binding sites and the adsorbate and a decrease in thickness of boundary layer of the adsorbent at high temperature. Besides the value of R², the applicability of kinetic models is verified through the sum of error squares (Lata et al., 2008) given by:

(7)

$$\mathit{SSE}\%=\frac{\sqrt{\mathrm{\Sigma }{(q_{e\text{,}\mathit{cal}}-q_{e\text{,}\exp })}^2}}{N}$$ where N is the number of data points.

Close

Figure 5

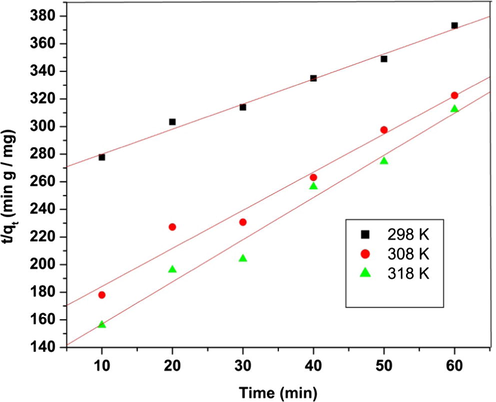
Pseudo-second order kinetic plot for adsorption of tartrazine over saw dust. Experimental condition: pH 3, adsorbent dosage 5 g/L, contact time 70 min.

Export to PPT

The higher value of R² and the lower value of SSE (Table 2) calculated in the case of pseudo second order kinetic model indicates better fitness of the data and indicates that the adsorption follows pseudo second order kinetics. A similar observation was reported for adsorption of tartrazine onto fly ash and a resin (Pura and Atun, 2009; Baraka, 2012). The calculated results are given in Table 3.

Table 3 Isotherm constants for the removal of Tartrazine on sawdust at different temperatures.

Adsorption isotherms and their constants	Temperatures (K)
	298	308	318
Langmuir adsorption isotherm
Kl (L g−1)	3.12	4.20	7.14
aL (L mg−1)	0.92	1.13	1.52
(mg g−1)	3.39	3.72	4.71
Rl	0.067	0.55	0.043
R2	0.974	0.977	0.982
Freundlich adsorption isotherm
Kf (mg g−1) (L mg−1)1/n	0.79	0.96	1.23
n	4.90	5.81	6.21
R2	0.974	0.965	0.954
Temkin adsorption isotherm
KT (L mg−1)	1.09	1.39	1.56
B	0.285	0.266	0.258
R	0.978	0.989	0.989
D–R adsorption isotherm
qm (mg g−1)	1.24	1.35	1.48
P (mol2 kJ−2)	0.051	0.039	0.031
E (kJ mol−1)	3.01	3.62	3.86
R2	0.986	0.997	0.997

Arrhenius (1889) proposed the relationship between the rate constant and temperature which could be described using the following expression (Zhang et al., 2011):

(8)

$$k_2=k_0{e}^{-E_a/\mathit{RT}}$$

This can be rearranged to linear relationship as:

(9)

$$\mathit{ln}{k}_2=\mathit{ln}{k}_0-\frac{E_a}{\mathit{RT}}$$ here k₂ is the rate constant of the pseudo second order adsorption kinetics rate, k₀ is the frequency factor, R is the gas constant (8.314 J/mol K) and E_a (kJ/mol) is the activation energy for the adsorption process. The magnitude of the activation energy can indicate the type of sorption. The activation energy was obtained from the slope of ln k₂ values versus 1/T using Eq. (10) and is found to be 19.79 kJ/mol.

(10)

$$\mathit{ln}{k}_2=-2385\frac{1}{T}+4.35(R^2=0.9642)$$

For adsorption processes with an activation energy less than 40 kJ/mol (Zhang et al., 2011), the main interaction between the dye and saw dust is probably physisorption.

3.5.4

3.5.4 Sorption mechanism

For proper corroboration of the adsorption process, the rate determining step is an important factor. Adsorption process is governed by either external mass transfer or intraparticle diffusion or both i.e. solute transfer processes. The overall rate of adsorption is controlled by either film diffusion or pore diffusion. It is clear from Fig. 6 that the adsorption process at the selected values of temperature has been divided into two phases. The initial curved portion of the plot indicates boundary layer effect while second linear portion due to pore diffusion. The biphasic nature of intraparticle diffusion plot confirms that both film and pore diffusion are involved in the process of dye removal. In order to differentiate between particle diffusion and film diffusion, Boyd kinetic expression (Boyd et al., 1947) was employed to the experimental data. Film diffusion occurs when external transport is greater than internal transport and in the case of particle diffusion internal transport is greater than external transport (Mittal et al., 2007).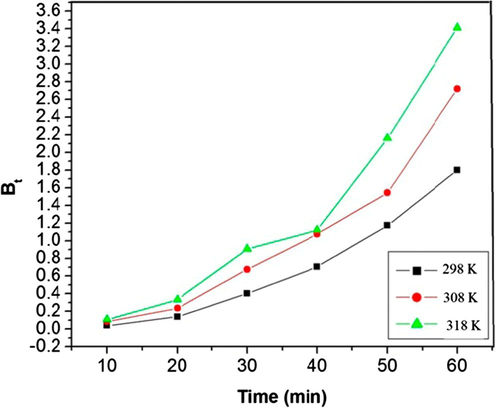

Close

Figure 6

Plot of B_t versus t (Boyd plot) at three different temperatures.

Export to PPT

A quantitative treatment of the sorption process can be investigated by using the following expressions:

(12)

$$F=1-\frac{6}{{\mathrm{\Pi }}^2}{\displaystyle \sum _1^{\infty }}\left(\frac{1}{n^2}\right)\exp (-n^2{B}_t)$$

(13)

$$F=\frac{q_t}{q_e}$$ Here q_e is the amount of dye adsorbed at infinite time (mg/g), q_t the amount of dye adsorbed at any time, F is the fraction of the solute adsorbed at any time t and B_t is mathematical fraction of F.

(14)

$$B=\frac{{\mathrm{\Pi }}^2}{r^2}{D}_i$$ where B is time constant and values of B were calculated from the slope of ‘B_t versus t’ plot, D_i is the effective diffusion coefficient of adsorbents and r represents radius of the particle (0.25 mm).

The F value can be used to determine the corresponding B_t values using the Richenberg’s table (Richenberg, 1953). Film diffusion and particle diffusion adsorption rate are determined with the help of B_t versus time plot (Fig. 6). The linearity of these plots was used to distinguish between film diffusion and pore diffusion controlled rates of adsorption. A straight line passing through the origin indicates that adsorption processes are governed by pore diffusion mechanisms for the present study. However it is observed from Fig. 6 that the relation between B_t and t is linear (average R² = 0.974), but does not pass through origin. This result confirms that surface diffusion is the rate limiting step where external transport of dye is more than internal transport. The values of effective diffusion coefficient D_i (cm² s⁻¹⁾ are calculated at 298 K, 308 K and 318 K and are summarized in Table 2. As reported earlier (Singh et al., 2011), in the case of intra particle diffusion or pore diffusion as a rate-limiting step in the adsorption process, a D_i value is of the order of (10 to 11) cm² s⁻¹. In this study, the average value of D_i obtained is 1.59 × 10⁻³ cm² s⁻¹, which is 7 orders of magnitude greater than the value quoted by Singh et al. This indicates that pore diffusion is not the rate limiting step. Similar results have been reported by Aravindhan et al. (2007) and Zou et al., 2011. The increasing values of effective diffusion coefficient D_i indicate that as temperature increases from 298 K to 318 K the mobility of ions rises due to the decrease of retarding force acting on diffusing ions of the dye.