Kinetic formation of iron oxide nanoparticles using un- and γ-irradiated singular molecular precursor of Tris(pentanedionato)iron(III) complex

3.1 Characterization of iron oxide nanoparticles and γ- irradiation role in its formation

The role of irradiation on the characteristic FTIR bands of tris(2,4-pentanedionato)iron(III) precursors were elucidated and shown in Fig. 1. As shown, the FTIR spectra of un-irradiated and γ-irradiated tris(2,4-pentanedionato)iron(III) with 100 and 300 kGy, respectively; in agreement with the literature (Slabzhennikov et al., 2003; Tayyari & Milani-nejad, 2000) displayed the characteristic bands assigned to various vibration modes of acetylacetonate functional groups with no disappearance or appearance of new bands as were recorded in the FTIR spectra of γ-irradiated tris(2,4-pentanedionato)iron(III) samples with 100 and 300 kGy total γ ray doses. However, a decrease in the intensity of most characteristic bands was recorded as results of γ-irradiation. The bands intensities designated to ν Fe-O bond in the range of 799 to 433 cm⁻¹ were more affected by irradiation than any other bands in the spectrum. The decreased in the intensity of this band could be attributed to bond divided or split influenced by γ irradiation.(Aly & Elembaby, 2020; El-Boraey et al., 2022) IR bands for tris(2,4-pentanedionato)iron(III) and their assignments are listed in Table 1.

Close

Fig. 1

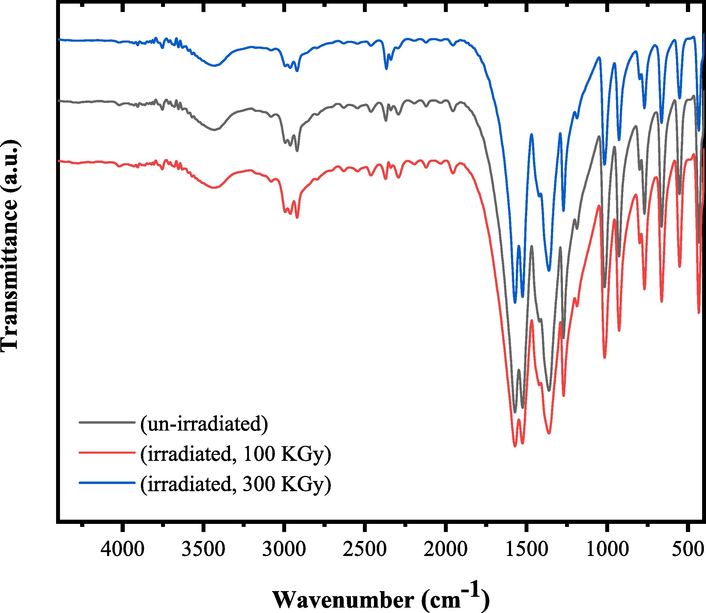
FTIR spectra of un-irradiated and γ irradiated tris(2,4-pentanedionato)iron(III) with 100 and 300 KGy, respectively.

Export to PPT

The role of irradiation on the XRD patterns of tris(2,4-pentanedionato)iron(III) precursors were also explored as shown in Fig. 2 which presented XRD patterns of un-irradiated and γ irradiated tris(2,4 pentanedionato)iron(III) with 100 and 300 kGy, respectively. The main peaks located at 10.99^◦ (0 2 0), 13.27^◦ (consisting of two superimposed peaks, 13.28^◦ (0 0 2) and 13.26^◦ (2 0 1)), 17.08^◦ (0 2 2), 21.54^◦ (3 2 1), 22.96^◦ (2 0 3), 23.95◦ (2 3 2) and 25.43◦ (2 2 3) matching a reference primitive orthorhombic phase (ICCD Card No: 00–030-1763) (Dudek et al., 2020). XRD diffractograms proved that orthorhombic tris(2,4 pentanedionato)iron(III) was only dominated phase in un-irradiated and γ irradiated samples and thus maintains its structural integrity after exposure to the irradiation dose. However, tris(2,4 pentanedionato)iron(III) exposure to the 300 kGy irradiation dose not only resulting in broadening of the main peaks but also appears a loss of peak intensity in addition to the 020 peak 2θ position specifically shift to 10.70°. This then demonstrates that irradiation doses with 300 kGy doses cause decreasing the degree of crystallinity and more damage to tris(2,4 pentanedionato)iron(III) than 100 kGy doses.

Close

Fig. 2

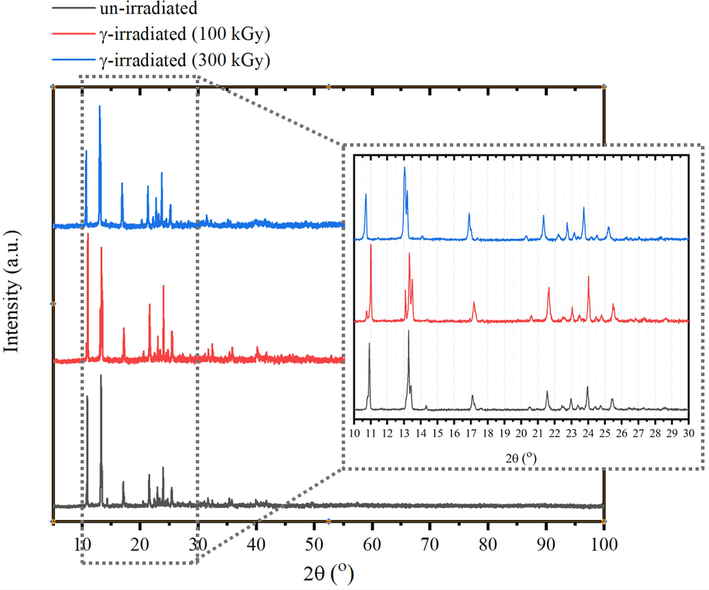
XRD patterns (a) of un-irradiated and γ irradiated tris(2,4 pentanedionato)iron(III) with 100 and 300 kGy, respectively.

Export to PPT

The irradiation effect on the morphology and surface elemental compositions of tris(2,4-pentanedionato)iron(III) precursors samples were examined as Fig. 3 displayed the effects of γ-ray on SEM images and EDS analysis of un-irradiated and γ irradiated tris(2,4 pentanedionato)iron(III) with 100 and 300 kGy samples. It can be clearly seen that the structure of inspected samples is ambiguous in nature, displaying a random size distribution for both un-irradiated and γ-irradiated samples containing of nearly spherical-shape nanoparticles that agglomerated together to form significant large grains that uniformly distributed throughout the matrix of the samples. The agglomeration of the grain crystallites increases with increasing the γ-irradiation with no discrete particles are noticeable in precursors samples. The EDS spectra revealed distinct signals at 0.25, 0.50, 0.57, and 6.40, corresponding to C, O, and Fe, respectively. Additionally, unassigned signals at 0.00 and 2.2 were identified, associated with C and Au. Tris(pentanedionato)iron(III) complexes exhibit minimal alterations when subjected to 100 and 300 kGy doses of gamma irradiation, as depicted in Fig. 4. Despite the application of high-energy photons inducing subtle changes evident in the resulting TGA curve, the complex remains remarkably stable. The intricate portrayal of the complex's thermal decomposition, leading to the formation of iron oxide and the subsequent release of byproducts, is clearly manifested in the TGA curve. The discernible impact of gamma irradiation on weight loss is characterized by slight shifts in the onset temperature of decomposition, fluctuations in the degradation rate, and modest alterations in the overall mass loss profile, reflecting the stability of the complex under the influence of gamma radiation. The TGA curves of un-irradiated tris(2,4 pentanedionato)iron(III) and γ-irradiated with 100 kGy displayed one major decomposition step with weight loss of 85.4 % in the range of 180 to 240 ℃ attributed to the thermolysis of pentanedionato moiety of the tris(2,4 pentanedionato)iron(III) phase resulting in the rapid formation of degradation products of gaseous residues and small iron oxide nuclei. The final weight of 14.6 % (calculated 21.8 %) is presumed to be the residue of the oxygen deficient iron oxide material. TGA curve demonstrates that γ-irradiated tris(2,4 pentanedionato)iron(III) with 100 kGy shows no apparent response to gamma irradiation doses and retains the thermolysis behaviour without any observed changes. Thermolysis of γ-irradiated tris(2,4 pentanedionato)iron(III) with 300 kGy rate dose exhibited similar behaviour with one major step with weight loss of 78.6 % in the range of 180 to 240 ℃ due to decomposition of pentanedionato moieties, and the final weight of 21.4 % (calculated 21.8 %) is presumed to be iron oxide products. The continuous weight loss on approaching 500 ℃ as consequence of decomposition of remaining carbonaceous species.

Close

Fig. 3

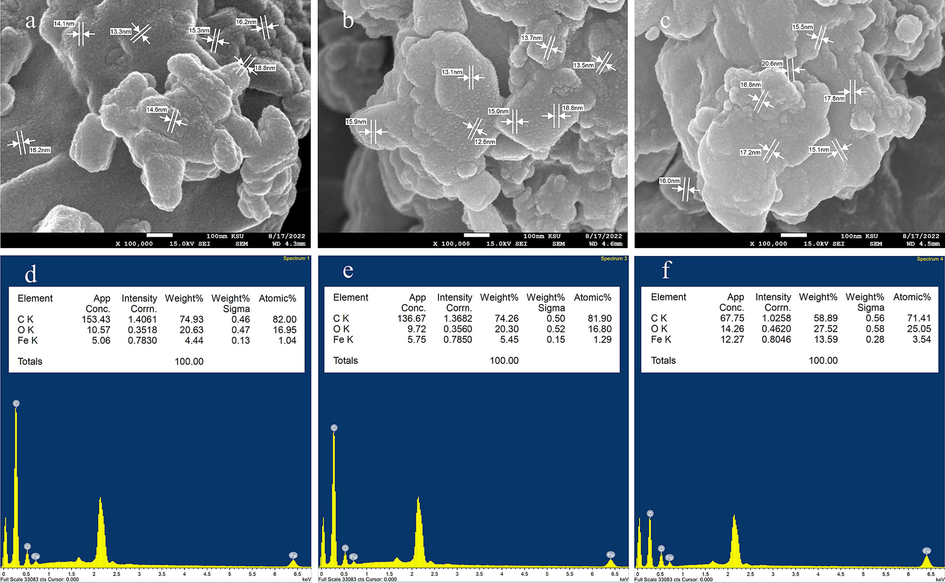
SEM/EDS analysis of un-irradiated (a and d) and γ irradiated tris(2,4 pentanedionato)iron(III) with 100 (b and e) and 300 kGy (c and f), respectively.

Export to PPT

Close

Fig. 4

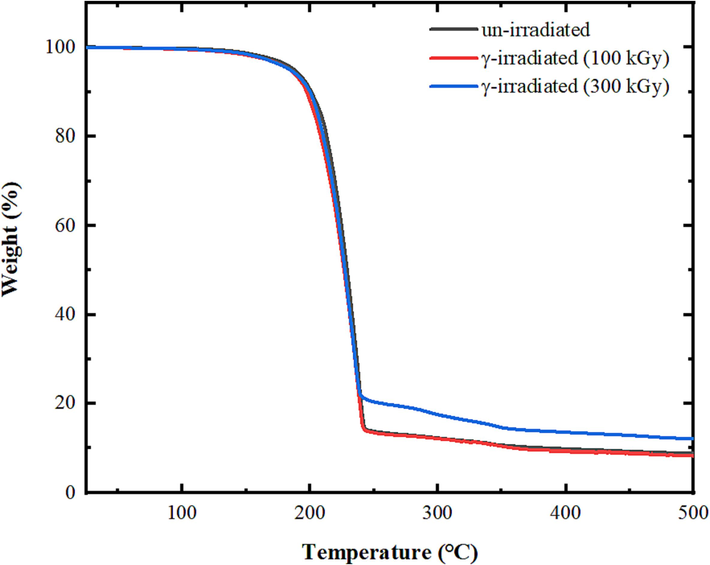
Effects of γ-ray on the thermal decomposition behaviour of tris(pentanedionato)iron(III) samples.

Export to PPT

The un-irradiated and γ irradiated tris(2,4 pentanedionato)iron(III) with 100 and 300 kGy, were calcined at selected temperature of 180, 200, 220, 240, 260, and 280 ℃ for 6 h each. Figs. 5 to 7 show the temperature-dependent FTIR spectra of un- and γ-irradiated calcined tris(2,4-pentanedionato)iron(III) with 100 and 300 kGy, respectively. The characteristic absorption bands of 2,4-pentanedionato gradually decrease with the increasing temperature and disappear when the temperature reaches 280 ℃, which indicates completion of the decomposition of 2,4-pentanedionato residues. The observed FTIR bands in the range of 800–––400 cm⁻¹, attributed to Fe-O vibrations, exhibit an intriguing behavior of increasing intensity with rising temperature. These bands are linked to the vibrational modes associated with specific iron oxide phases, and their variations at different temperatures provide valuable insights into the thermal evolution of the formed iron oxide. At 240 °C, the presence of bands at 660, 614, 550, 446, and 428 cm⁻¹ aligns with the characteristic vibrational modes of magnetite (Fe₃O₄). The increased intensity of these bands at higher temperatures may indicate enhanced crystallinity or phase transitions within the magnetite structure. Upon further heating to 260 °C, additional bands at 728, 692, 640, 560, 480, 444, and 428 cm⁻¹ emerge. These bands are associated with vibrational modes characteristic of maghemite (γ-Fe₂O₃). The growing intensity of these bands suggests a temperature-induced transformation towards maghemite, possibly indicating the conversion from magnetite to maghemite. At 280 °C, the vibrational bands at 728, 697, 640, 560, 484, 444, and 424 cm⁻¹ remain, affirming the persistence of maghemite characteristics. The variations in band intensity across temperatures imply dynamic phase changes and the influence of thermal treatment on the iron oxide system. However, it's important to note that the identification of phases based on FTIR bands is indicative, and definitive confirmation often requires complementary techniques such as X-ray diffraction (XRD). The observed changes underscore the sensitivity of FTIR spectroscopy to alterations in the crystalline structure and phase composition of iron oxides under different thermal conditions (Stoia et al., 2016; Waldron, 1955; Yadav et al., 2020). Moreover, the observed broad band at 3401 cm⁻¹ and band at 1624 cm⁻¹ were attributed to both symmetrical and unsymmetrical modes of O–H bonds of adsorbed water layer associated to the surface iron atoms. The temperature-dependent FTIR spectra of γ-irradiated calcined tris(2,4-pentanedionato)iron(III) with 100 and 300 kGy as seen in demonstrated similar behaviour with decreasing intensities of the iron oxide characteristic absorption bands for the calcined sample of tris(2,4-pentanedionato)iron(III) precursor with 300 kGy sample related to the calcined un-irradiated sample of tris(2,4-pentanedionato)iron(III) precursor.

Close

Fig. 5

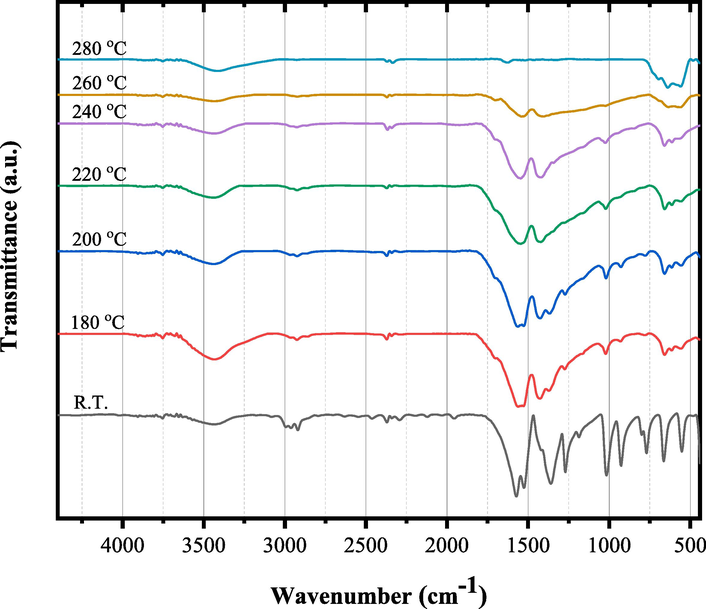
FT-IR spectra of un-irradiated tris(2,4-pentanedionato)iron(III) calcined at different temperatures for 6 h.

Export to PPT

Close

Fig. 6

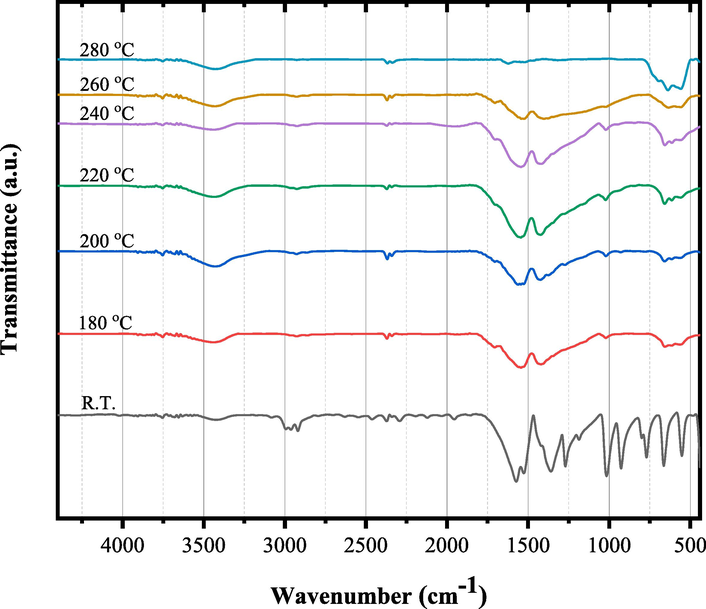
FT-IR spectra of γ-irradiated tris(2,4-pentanedionato)iron(III) with 100 kGy radiation dose calcined at different temperatures for 6 h.

Export to PPT

Close

Fig. 7

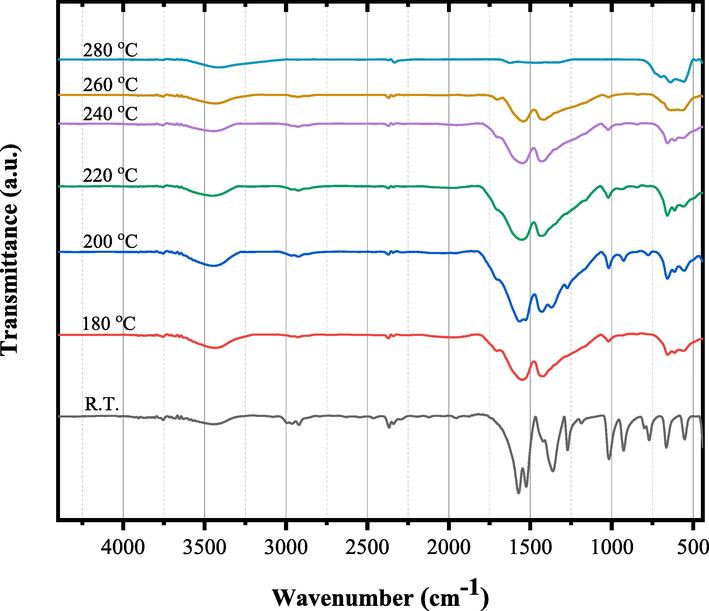
FT-IR spectra of γ-irradiated tris(2,4-pentanedionato)iron(III) with 300 kGy radiation dose calcined at different temperatures for 6 h.

Export to PPT

Figs. 8–10 show the temperature-dependent XRD patterns of un- and γ-irradiated calcined tris(2,4-pentanedionato)iron(III) with 100 and 300 kGy, respectively. Through calcination at 180, 200, 220, 240, 260, and 280 ℃ for 6 h each, an immediate structural loss of the corresponding tris(2,4-pentanedionato)iron(III) precursor was observed accompanied by the emission of carbonous gases from 2,4-pentanedionato moieties decomposition resulting in the formation of amorphous phases contain iron oxide. Moreover, the reflection signal located at 2θ of 36° in the diffractogram of calcined sample at 220 ℃ started to appear signalling the formation of iron oxide material phase. Upon increasing of calcination temperatures reaching 280 ℃ as shown in Fig. 11, All the reflections in the XRD patterns can be indexed to Magnetite iron oxide phase (ICCD Card No: 00–003-0863). The characteristic peaks at 30.4°, 35.8°, 43.5°, 53.9°, 57.4°, and 63.2° match to the (2 2 0), (3 1 1), (4 0 0), (4 2 2), (5 1 1), and (4 4 0) crystal faces of Fe₃O₄, respectively. The signals get well defined and narrowed indicating the effect of pyrolysis temperatures on the XRD patterns in alignment with the FTIR and TGA analyses conforming of nanocrystalline iron oxide phase developments. The peaks are found at 12.77° can be attributed to the amorphous phase of carbon or to deletion of some specific interlayered atoms leads to increased intensities.(Sun et al., 2017) The crystallite size of the prepared iron oxide samples was calculated by averaging the values obtained from the (2 2 0), (3 1 1), (4 0 0), (4 2 2), (5 1 1), and (4 4 0) diffraction peaks, utilizing the Debye-Scherrer equation (Eq. (1):

Close

Fig. 8

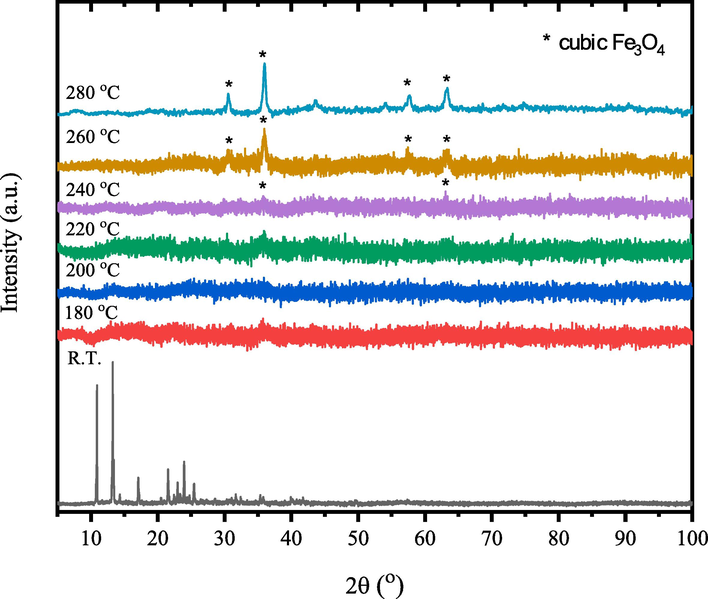
Temperature-dependent XRD patterns of the un-irradiated tris(2,4-pentanedionato)iron(III).

Export to PPT

Close

Fig. 9

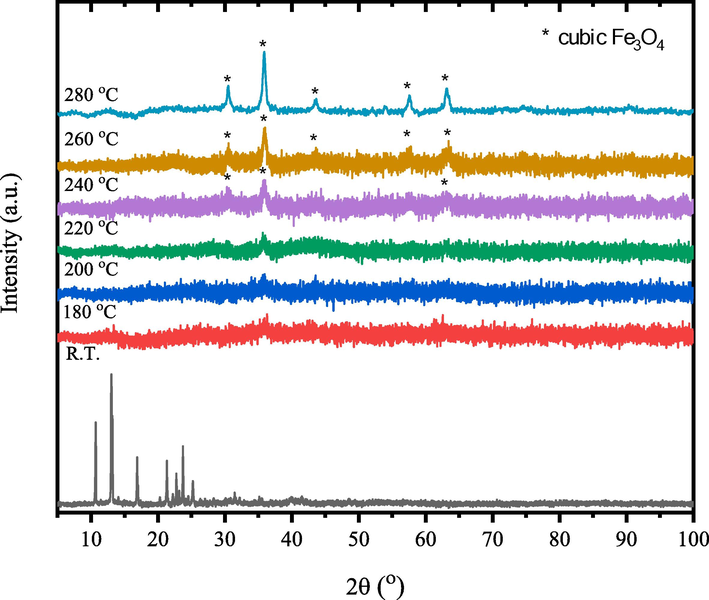
XRD patterns of γ-irradiated tris(2,4-pentanedionato)iron(III) with 100 kGy radiation dose calcined products at different temperatures for 6 h.

Export to PPT

Close

Fig. 10

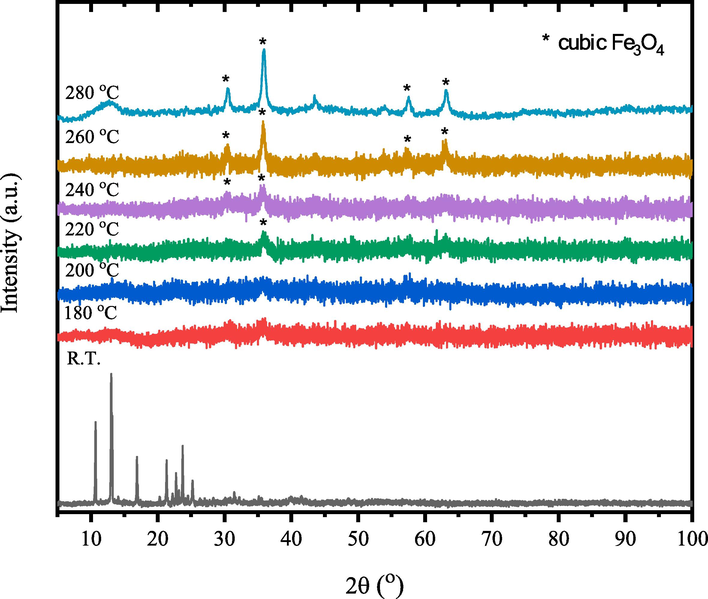
XRD patterns of γ-irradiated tris(2,4-pentanedionato)iron(III) with 300 kGy radiation dose calcined products at different temperatures for 6 h.

Export to PPT

Close

Fig. 11

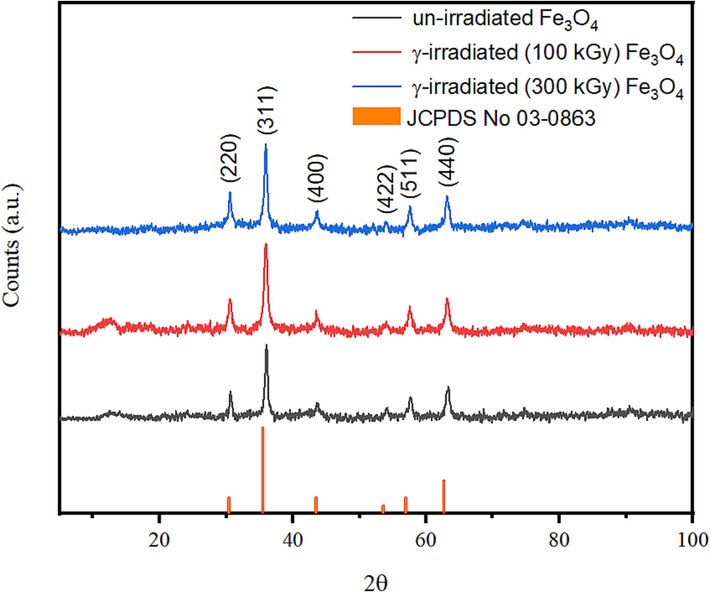
XRD patterns of the solid residues obtained from pyrolysis of un-irradiated and γ-irradiated tris(2,4-pentanedionato)iron(III) precursors with 100 and 300 kGy, respectively.

Export to PPT

Where, D is the diameter of crystalline size, λ is the wavelength of the incident beam, β is the full width half maxim ‘FWHM’, and θ is the Bragg’s angle. The crystallite size as Table 2 demonstrated represents the size of the individual crystalline domains within the nanoparticles. In the un-irradiated sample of iron oxide samples obtained from pyrolysis, the crystallite size is 18.23 ± 0.23 nm, which decreases to 16.42 ± 0.21 nm and 13.964 ± 0.11 nm for the 200 kGy and 300 kGy irradiated samples, respectively. The reduction in crystallite size with increased irradiation suggests a transformation in the crystal structure, potentially due to radiation-induced defects or phase changes. Fig. 12 clearly indicated that the as-prepared iron oxide materials were nearly spherical-shape nanoparticles with average size of 28.65 ± 4.08, 27.35 ± 6.14, and 22.99 ± 4.46 nm for samples obtained from pyrolysis of un-irradiated and γ-irradiated tris(2,4-pentanedionato)iron(III) precursors with 100 and 300 kGy, respectively (Table 2). The particles agglomerated together, and elements distributed uniformly all over the matrix of the samples. These results confirmed that the corresponding oxides kept the spherical morphology with a broader size distribution of the apparent original precursors. The agglomeration of the grain crystallites increases, and the particle size decreases with increasing the γ-irradiation. Pore size and BET surface area are vital indicators of the nanoparticles' structural characteristics (Figure S4 in SF Text). In the un-irradiated sample, the pore radius is 37.651 Å, while the BET surface area is 110.909 m²/g. With irradiation, the pore radius decreases to 23.152 Å (200 kGy) and 23.294 Å (300 kGy), and the BET surface area increases to 121.352 m²/g (200 kGy) and 153.991 m²/g (300 kGy). The reduction in pore radius and increase in BET surface area suggest enhanced porosity and surface reactivity, possibly attributable to the radiation-induced structural modifications. The dynamic changes in crystallite size, pore size, and BET surface area highlight the influence of irradiation on the physical properties of the nanoparticles. These alterations are pivotal for tailoring nanoparticles to potential specific applications, whether in catalysis, sensing, or drug delivery, and underscore the importance of a comprehensive understanding of their evolving characteristics under different irradiation conditions. Fig. 12 also demonstrate the EDS analysis of un-irradiated and γ irradiated of the solid residues obtained from pyrolysis of tris(2,4-pentanedionato)iron(III) precursors with 100 and 300 kGy, respectively. The un-irradiated sample showcases weight percentages of 33.43 % oxygen (O) and 66.57 % iron (Fe). The calculated Fe/O ratio approximates 2, indicating a molecular formula close to O₂Fe₁. This aligns with the expected composition of iron oxide, potentially magnetite, emphasizing the dominant role of iron in the molecular structure. Upon irradiation with 100 kGy, a shift in weight percentages to 30.87 % O and 69.13 % Fe alters the Fe/O ratio to approximately 2.24, suggesting a modified molecular formula of O₁.₉Fe₁.₂. The increased iron content implies potential phase transformations induced by irradiation, influencing the material's composition. At 300 kGy, weight percentages further change to 23.71 % O and 76.29 % Fe. The Fe/O ratio increases to about 3.21, indicating a shift towards O₁.₄ Fe₁.₃. This substantial alteration suggests a more pronounced impact of irradiation, potentially leading to the formation of iron-rich phases. The observed trends in molecular formulas and Fe/O ratios imply a dynamic response to radiation. The increasing Fe/O ratio suggests progressive iron enrichment, possibly indicating the formation of iron oxides with higher irradiation doses. Acknowledging the simplified nature of these estimations, further characterization techniques like Mössbauer spectroscopy are essential for precise phase identification. Overall, the EDS data illuminates the evolving molecular composition and Fe/O ratios, offering valuable insights into irradiation's impact on the material's structure and potential phase transformations.

Close

Fig. 12

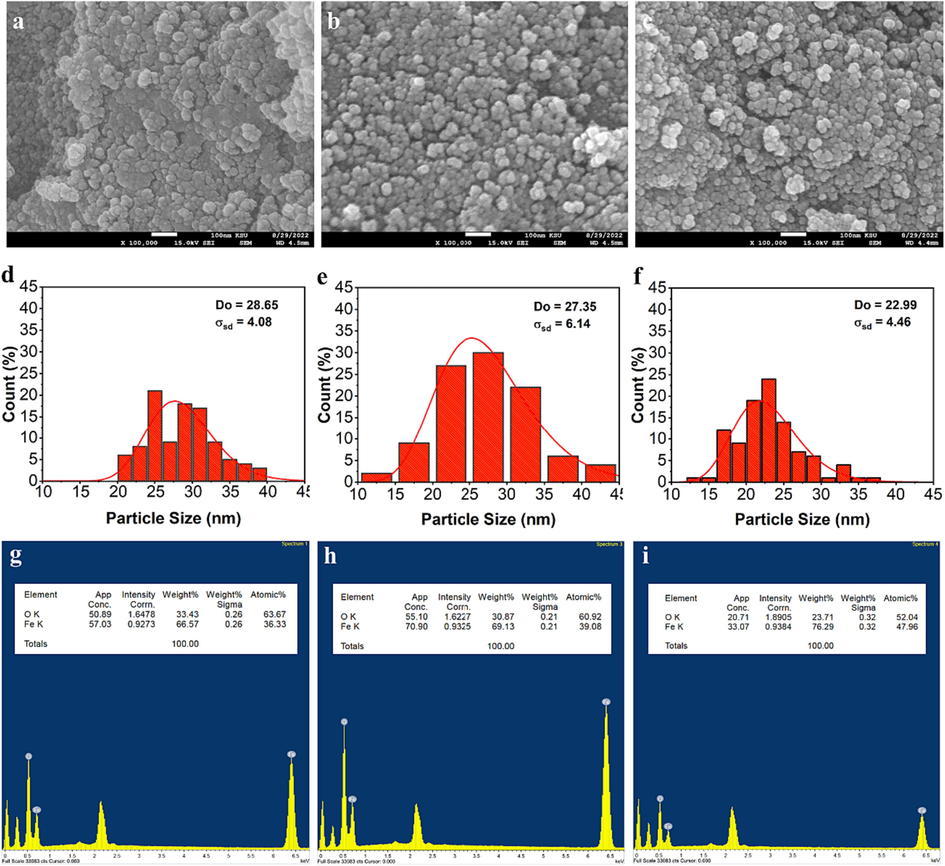
SEM image, particle size distribution histogram, and EDS analysis of un-irradiated (a, d and g) and γ irradiated of the solid residues obtained from pyrolysis of tris(2,4-pentanedionato)iron(III) precursors with 100 (b, e and h) and 300 kGy (c, f and i), respectively.

Export to PPT

3.2 Kinetic Studies

The solid-state decomposition reactions of tris(pentanedionato) iron (III) can be depicted through with a single-step kinetic equation according to Eq. (2).

Where $\frac{\mathrm{d}\alpha }{\mathrm{d}t}$ is the reaction rate, $t$ (min⁻¹) is the time, $k\left(T\right)$ is the rate constant depending on temperature, $T$ (K) is the absolute temperature, $f\left(\alpha \right)$ is the reaction model, and $\alpha$ is the degree of conversion which calculated via $\alpha =\frac{m_{°}-m}{m_{°}-m_{\infty }}$ ; $m_{°}$ (mg) is the initial weight of the sample, $m$ (mg) is the weight of the sample at temperature $T$ , and $m_{\infty }$ (mg) is the final weight of the sample. (Galwey & Brown, 1998; Reading, 1998; Sharp et al., 1966; van Ekeren, 1998). The Arrhenius equation is applied to explicitly integrate the temperature dependence into the rate constant (Eq. (3):

In this expression, A represents the pre-exponential factor (min⁻¹), $E_a$ is the activation energy (kJmol⁻¹), and R signifies the gas constant. The amalgamation of Arrhenius parameters and the reaction model is commonly referred to as the kinetic triplet ( $E_a$ , $A$ , and $f\left(\alpha \right)$ ). In situations involving nonisothermal conditions, where a sample undergoes constant-rate heating, the explicit temporal dependence in Eq. (3) is eliminated through a straightforward transformation (Eq. (4):

Here, $\frac{\mathrm{d}T}{\mathrm{d}t}=\beta$ denotes the heating rate. Fig. 13 examines the influence of heating rate on the thermal decomposition of un- and γ-irradiated tris(pentanedionato) iron (III) samples materials. Fig. 13 illustrates that higher heating rates shift the reaction zone towards elevated temperatures. This phenomenon results from the varied residence times of tris(pentanedionato) iron (III) samples under different heating rates. The relationship between heating rates and residence time is inversely proportional; lower heating rates correspond to longer residence times, allowing thermal gradients to penetrate the inner core of sample particles. The kinetic triplet parameters for each decomposition step can be derived by rearranging and integrating the non-isothermal rate law from Eqs. (4) and (5), employing both model-fitting and isoconversional approximations.

Close

Fig. 13

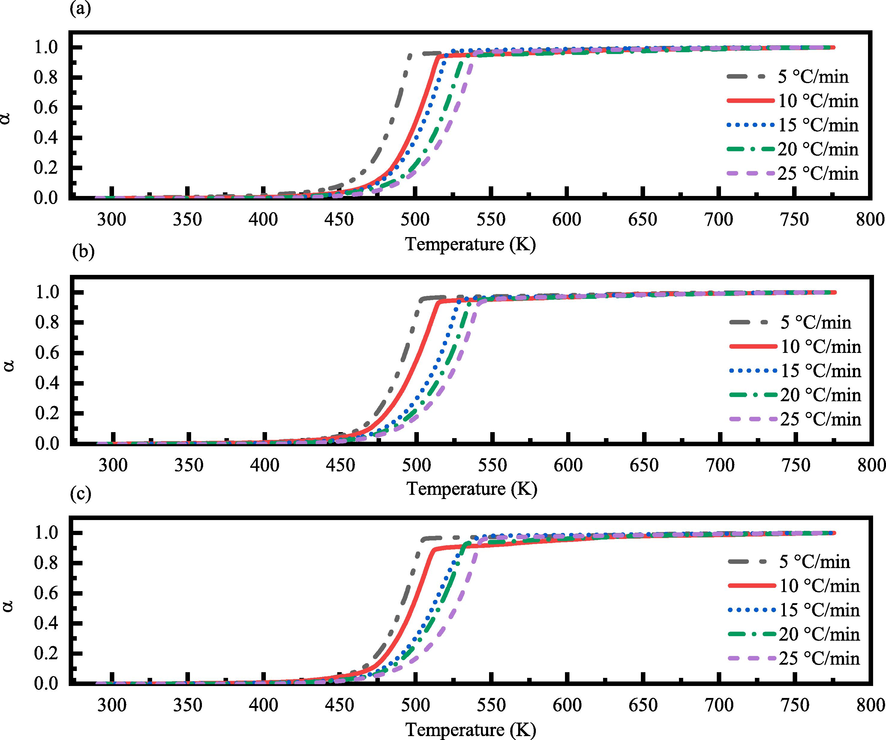
α-T (K) curves of the thermal decomposition for (a) un-irradiated, (b) γ-irradiated with 100 kGy radiation dose, and (c) γ-irradiated with 300 kGy radiation dose of tris(pentanedionato) iron (III) samples in static air at different heating rates.

Export to PPT

Where $P\left(x\right)={\int }_0^{\alpha }{[f(\alpha )]}^{-1}\mathrm{d}\alpha$ is the exponential integral which has numerous approximations and no direct analytical solution. (Brown, 1997; Galwey & Brown, 2000; Khawam & Flanagan, 2005; Vyazovkin, 2000).

3.2.1

3.2.1 Model-fitting approaches

The solid-state reaction model equations, Table A in SF Text, employed into experimental data to obtain the kinetic parameters through model-fitting approaches using Coates and Redfern (CR) and Clark and Kennedy (CK) model displayed in Eqs. (6) and (7). (Coats & Redfern, 1964; Kennedy & Clark, 1997; Vyazovkin, 2000; Vyazovkin & Wight, 1999a)

(6)

$$\mathrm{l}\mathrm{n}\frac{g(\alpha )}{T^2}=\mathrm{l}\mathrm{n}\left(\frac{\mathit{AR}}{\beta E_a}\left[1-\left(\frac{{2RT}^{\ast }}{\beta E_a}\right)\right]\right)-\frac{E_a}{\mathit{RT}}$$

(7)

$$\mathrm{l}\mathrm{n}\frac{\beta g(\alpha )}{T-T^{°}}=\mathrm{l}\mathrm{n}A-\frac{E_a}{\mathit{RT}}$$

Where $T^{\ast }$ and $T^{°}$ is the mean and initial temperatures, respectively. Kinetic parameters for un-irradiated and γ-irradiated tris(2,4-pentanedionato)iron(III) with 100 and 300 kGy are obtained by plotting the left-hand side of both equations versus the inverted temperatures. (Rodante et al., 2002) From the slope and intercept of CR and CK equations the values of $E_a$ , $A$ , and r were obtained (Tables B to G in SF Text). The function model with best linear of temperature dependency to evaluate the reaction rate constant k(T) found to be adapting the two-dimensional diffusion (bi-dimensional particle shape) Valensi equation (D₂) as shown in Fig. 14 for all samples.

Close

Fig. 14

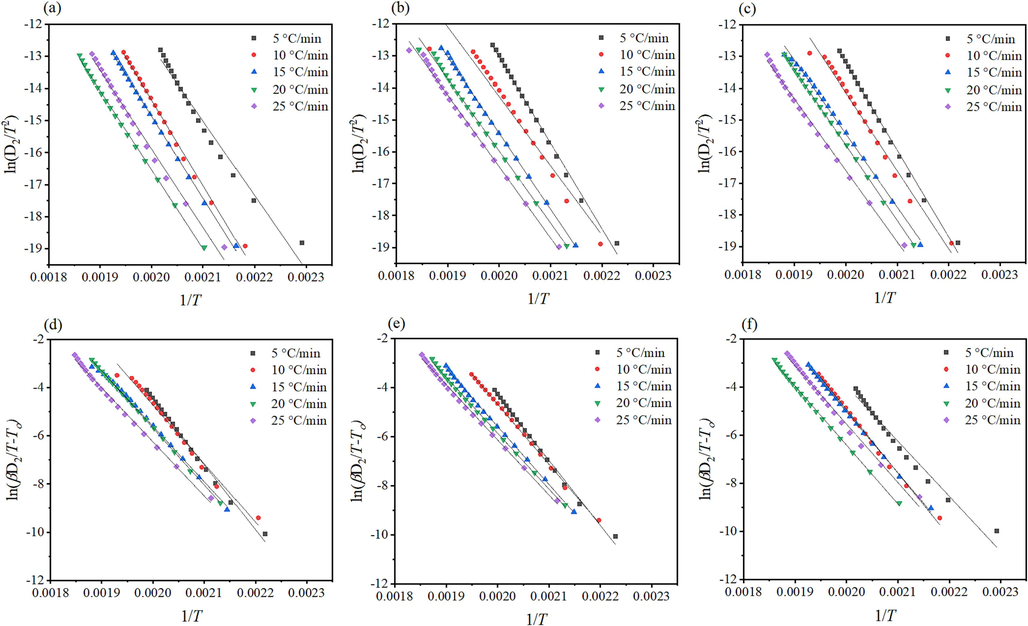
Kinetic analysis of α/T (K) curves of the thermal decomposition of tris(pentanedionato) iron (III) samples according to CR (top) and CK (bottom) for (a and d) un-irradiated, (b and e) γ-irradiated with 100 kGy, and (c and f) γ-irradiated with 300 kGy radiation dose.

Export to PPT

3.2.2

3.2.2 Isoconversional approaches

In nonisothermal experiments, the difficulty lies in distinguishing temperature dependence k(T) from the reaction model f(α), resulting in uncertainties in Arrhenius parameters that compensate for disparities between assumed and actual reaction models. Model-fitting provides a single, averaged activation energy, overlooking variations with temperature and conversion extent. Isoconversional methods address these challenges by determining activation energy without assuming the reaction model, thereby providing more consistent kinetic results across experiments. (Vyazovkin & Wight, 1999b) Isoconversional approaches were utilized to estimate the relation between activation energy ( $E_a$ ) and the degree of conversion ( $\alpha$ ) on dependency of the temperature (T) based on model-free assumptions. (Khawam & Flanagan, 2005; Sbirrazzuoli & Vyazovkin, 2002) Several approaches, include Kissinger-Akahira-Sunose (KAS), Tang (T), and Flynn-Wall-Ozawa (FWO) equations as Eqs. (8)–(10) demonstrated (Flynn & Wall, 1966; Kissinger, 1957; Tang & Chaudhri, 1980), were employed by plotting the left-hand sides against the inverted temperatures which gave a straight line at each value of $\alpha$ as Fig. 15 illustrated, and from the slope and intercept of each lines the activation energy were deduced (Table H in SF Text).

(8)

$$\mathrm{l}\mathrm{n}\beta =\ln \left(\frac{{\mathit{AE}}_a}{\mathit{Rg}(\alpha )}\right)-5.3305-1.052\left(\frac{E_a}{\mathit{RT}}\right)$$

(9)

$$\ln \left(\frac{\beta }{T^2}\right)=\mathrm{l}\mathrm{n}\frac{\mathit{AR}}{E_{a}g(\alpha )}-\frac{E_a}{\mathit{RT}}$$

(10)

$$\ln \left(\frac{\beta }{T^{1.894661}}\right)=\ln \left[\frac{{\mathit{AE}}_a}{\mathit{Rg}(\alpha )}\right]+3.635041-1.894661\ln E_a-1.001450\frac{E_a}{\mathit{RT}}$$

Close

Fig. 15

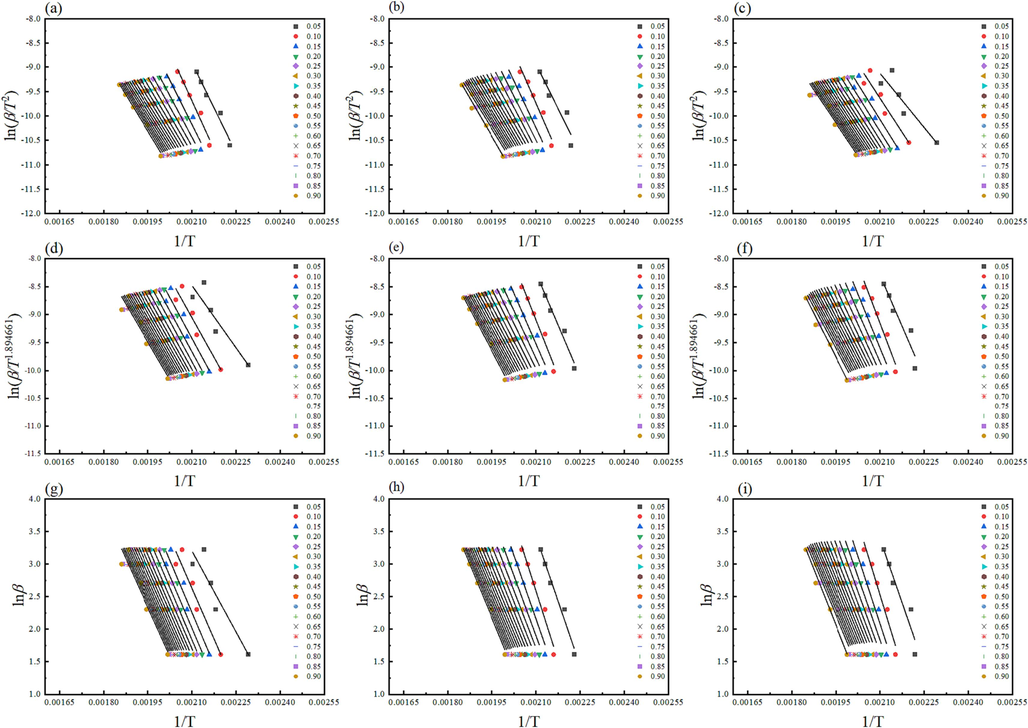
Kinetic analysis of the thermal decomposition of tris(pentanedionato)iron (III) samples according to KAS (top), T (middle), and FWO (bottom) for (a, d, and g) un-irradiated, (b, e, and h) γ-irradiated with 100 kGy, and (c, f, and i) γ-irradiated with 300 kGy radiation dose.

Export to PPT

Further, Vyazovkin (VYZ) isoconversional approximation with 5th degree Senum-Yang approximation (Eqs. (11) and (12) was used by Gorbachev (Eq. (13), Agrwal (Eq. (14), and Sivaubramanin-Cai (Eq. (15) integrations to determine the apparent activation energy ( $E_{a,\alpha }$ ) at any specific value of the extent of the conversion $(\alpha )$ in which the value of $\mathrm{\Omega }$ is minimized.(Saha & Ghoshal, 2006; Saha et al., 2006; Vyazovkin, 2000; Vyazovkin & Wight, 1997, 1999a) Fig. 16 displays similar dependency of $E_{a,\alpha }$ as a function of $\alpha$ using different isoconversional approximations for un-irradiated and γ-irradiated with 100 and 300 kGy radiation doses. Moreover, the $E_{a,\alpha }$ values of γ-irradiated tris(pentanedionato)iron(III) with 100 and 300 kGy radiation doses were increased in comparison to un-irradiated sample could be relay on reduction of active nucleation sites as role of irradiation during the decomposition reaction. The process assumed to be single step initially started with increasing activation energy with increasing the extant of conversion due to decomposition of organic residues and the formation of active nucleation centres of iron oxide. Furthermore, the $E_{a,\alpha }$ values differences attributed to temperature integral approximations that were used in base-relations derivations of KAS, T, FWO, VAS equations.

(11)

$$\mathrm{\Omega }=\left|\sum _{i=1}^n\sum _{i\ne j}^n\frac{I\left(E_{a,\alpha },T_{\alpha ,i}\right){\beta }_j}{I\left(E_{a,\alpha },T_{\alpha ,j}\right){\beta }_i}\right|$$

(12)

$$I\left(E_{a,\alpha },T_{\alpha }\right)={\int }_0^{T_{\alpha i}}\mathrm{e}\mathrm{x}\mathrm{p}\left(\frac{{-E}_{a,\alpha }}{\mathit{RT}}\right)\mathrm{d}T$$

(13)

$${\int }_0^T\mathrm{e}\mathrm{x}\mathrm{p}\left(\frac{{-E}_{\alpha }}{{\mathit{RT}}_{\alpha i}}\right)\mathrm{d}T=\frac{R{T}_{\alpha i}^2}{E_{\alpha }}\left(\frac{1}{1+\frac{2RT}{E_{\alpha }}}\right)\mathrm{e}\mathrm{x}\mathrm{p}\left(\frac{{-E}_{\alpha }}{{\mathit{RT}}_{\alpha i}}\right)$$

(14)

$${\int }_0^T\mathrm{e}\mathrm{x}\mathrm{p}\left(\frac{{-E}_{\alpha }}{{\mathit{RT}}_{\alpha i}}\right)\mathrm{d}T=\frac{R{T}_{\alpha i}^2}{E_{\alpha }}\left[\frac{1-\frac{2RT}{E_{\alpha }}}{1-5{\left(\frac{\mathit{ER}}{E_{\alpha }}\right)}^2}\right]\mathrm{e}\mathrm{x}\mathrm{p}\left(\frac{{-E}_{\alpha }}{{\mathit{RT}}_{\alpha i}}\right)$$

(15)

$${\int }_0^T\mathrm{e}\mathrm{x}\mathrm{p}\left(\frac{{-E}_{\alpha }}{{\mathit{RT}}_{\alpha i}}\right)\mathrm{d}T=\frac{R{T}_{\alpha i}^2}{E_{\alpha }}\left[\frac{\frac{E_{\alpha }}{{\mathit{RT}}_{\alpha i}}+0.66691}{\frac{E_{\alpha }}{{\mathit{RT}}_{\alpha i}}+2.64943}\right]\mathrm{e}\mathrm{x}\mathrm{p}\left(\frac{{-E}_{\alpha }}{{\mathit{RT}}_{\alpha i}}\right)$$

Close

Fig. 16

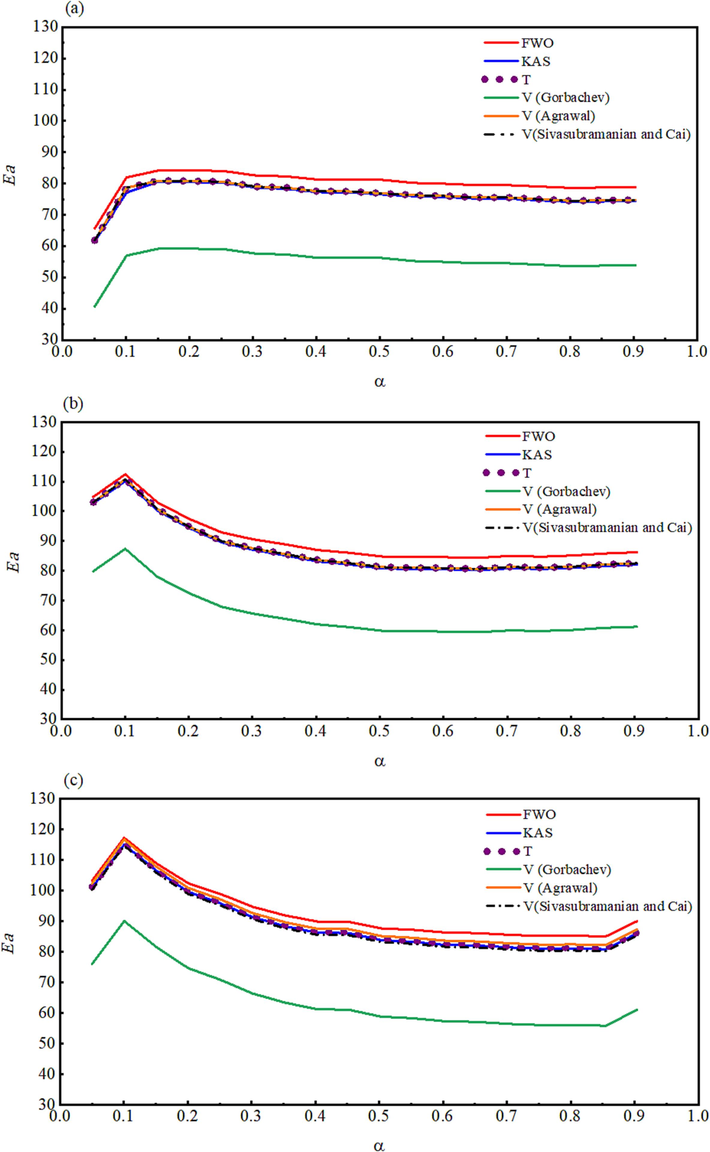
The activation energy plotted as a function of the extent of the conversion for (a) un-irradiated, (b) γ-irradiated with 100 kGy, and (c) γ-irradiated with 300 kGy radiation doses.

Export to PPT

3.2.3

3.2.3 Compensation effect

The validity of the compensation effect is related to the linearity of the relationship between lnA and $E_a$ for related chemical processes under different conditions utilizing various reaction models to the same set of nonisothermal kinetic data. The compensation effect is associated to artificial isokinetic relationship (IKR) when chemical reactions become identical at a certain isokinetic temperature T_iso which can be ascribed by Eq. (16):

(16)

$$\ln A_j=a+b{E}_{a,j}$$

Where j represents one of the possible models f_j(α) assumed to illustrate the reaction processes, $a$ and $b$ are constants corresponding to lnk_iso and 1/RT_iso, respectively. (Agrawal, 1986; Barrie, 2012; Bligaard et al., 2003; Cremer, 1955) Fig. 17 shows the artificial isokinetic relationship for the solid-state decomposition reactions of the un-irradiated and γ-irradiated tris(pentanedionato) iron (III) obtained by CK methods. The values of $a$ , $b$ , k_iso, T_iso of Eq. (16) attained by (CR and CK) model-fitting are given in Table 3. As shown the T_iso lie on experimental temperature region demonstrating that the reaction model f_j(α) was most likely selected. To estimate lnA_α and illustrate it dependency on α for the un-irradiated and γ-irradiated tris(pentanedionato) iron (III) decomposition process, the $E_{a,\alpha }$ value were exchanged for $E_{a,j}$ in Eq. (16).(Al-Othman et al., 2009; Vyazovkin & Wight, 1999a) Fig. 18 shows lnA_α relationship with α depicted form the applied isoconversional methods data for the decomposition processes of the un-irradiated and γ-irradiated tris(pentanedionato) iron (III) precursors with 100 and 300 kGy total dose ray, respectively. As exhibited, the lnA_α dependency on the extent of the conversion (α) is quite similar with the apparent activation energy ( $E_{a,\alpha }$ ) dependency on α in Fig. 16 supporting that the solid-state decomposition reaction of the un-irradiated and γ-irradiated tris(pentanedionato) iron (III) precursors presumed to be a single step initially started with increasing $E_{a,\alpha }$ and lnA_α with increasing the extant of conversion due to decomposition of organic moieties and the formation of active nucleation centres of iron oxide, and with 100 and 300 kGy radiation doses their values increased in comparison to un-irradiated sample attributed to the reduction of active nucleation sites during the decomposition reaction.

Close

Fig. 17

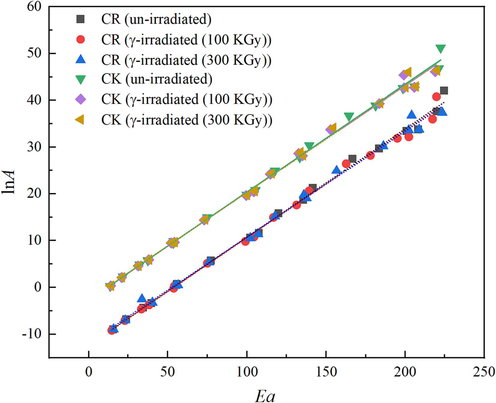
The isokinetic relationships obtain by CR and CK methods for un-irradiated and γ- irradiated tris(pentanedionato)iron(III) samples.

Export to PPT

Table 3 Artificial isokinetic parameter obtained by CR and CK methods.

Method	$a$ (min−1)	$b$ (mol KJ−1)	kiso (min−1)	Tiso (K)	r2
CR (un-irradiated)	−12.4080	0.2315	4.09 × 10-6	519.5639	0.9966
CR (γ-irradiated (100 kGy))	−12.4020	0.2298	4.11 × 10-6	523.4075	0.9959
CR (γ-irradiated (300 kGy))	−11.9180	0.2265	6.67 × 10-6	531.0333	0.9957
CK (un-irradiated)	−2.7136	0.2307	6.63 × 10-2	521.3656	0.9962
CK (γ-irradiated (100 kGy))	−2.5951	0.2287	7.46 × 10-2	525.9250	0.9965
CK (γ-irradiated (300 kGy))	−2.6055	0.2287	7.39 × 10-2	525.9250	0.9965

Close

Fig. 18

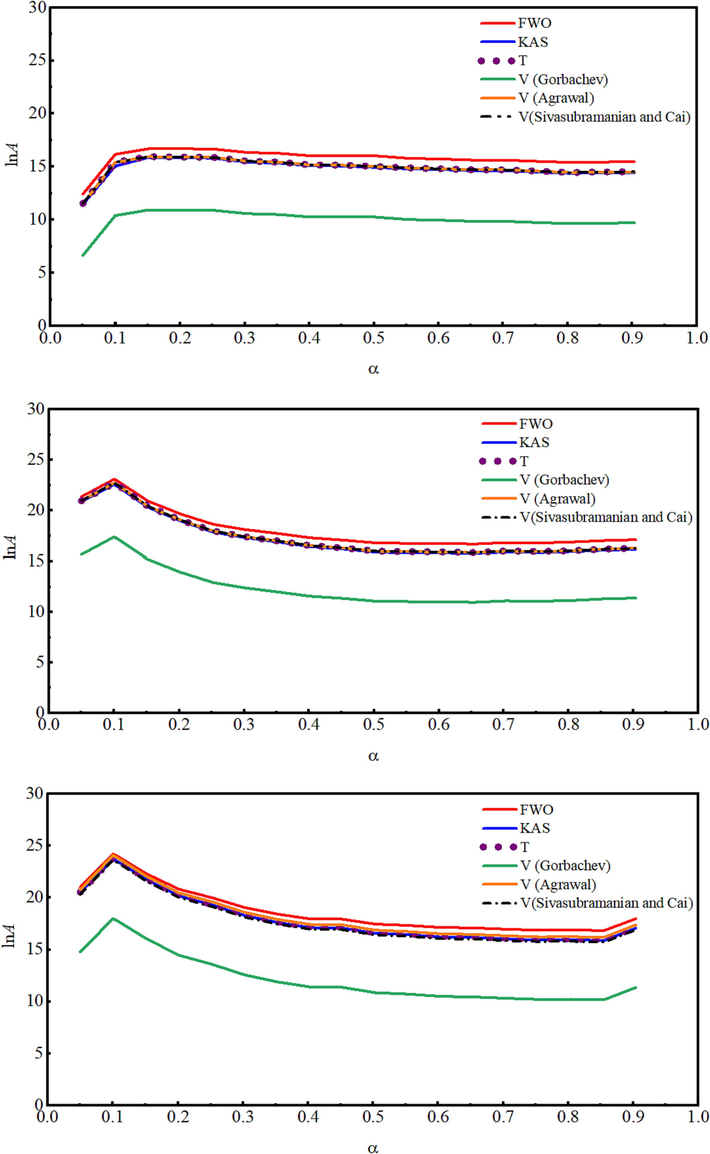
The lnA dependence on the extent of the conversion for (a) un-irradiated, (b) γ-irradiated with 100 kGy, and (c) γ-irradiated with 300 kGy radiation doses.

Export to PPT