Oxidation of aliphatic aldehydes by benzimidazolium fluorochromate in non aqueous medium – A kinetic and mechanistic study

Basim H. Asghar; S. Sheik Mansoor; A. Mohamed Hussain; V. Saleem Malik; K. Aswin; S.P.N. Sudhan

doi:10.1016/j.arabjc.2013.07.043

View/Download PDF

Buy Reprints

PDF

Translate this page into:

Original article

10 (

2_suppl

); S2115-S2123

doi:

10.1016/j.arabjc.2013.07.043

Oxidation of aliphatic aldehydes by benzimidazolium fluorochromate in non aqueous medium – A kinetic and mechanistic study

Basim H. Asghar^{^a}, S. Sheik Mansoor^{^b,⁎}, A. Mohamed Hussain^{^b}, V. Saleem Malik^{^b}, K. Aswin^{^b}, S.P.N. Sudhan^{^b}

a Department of Chemistry, Faculty of Applied Sciences, Umm Al-Qura University, P.O. Box 9569, Makkah, Saudi Arabia

b Research Department of Chemistry, C. Abdul Hakeem College, Melvisharam, 632 509 Tamil Nadu, India

⁎Corresponding author. Tel.: +91 9944093020; fax: +91 4172 266484. smansoors2000@yahoo.co.in (S. Sheik Mansoor)

Received: 2012-1-12, Accepted: 2013-7-21, Published: 2013-05-01

Disclaimer:
This article was originally published by Elsevier and was migrated to Scientific Scholar after the change of Publisher.

Peer review under responsibility of King Saud University.

Abstract

The oxidation of seven aliphatic aldehydes by benzimidazolium fluorochromate (BIFC) in dimethyl sulphoxide (DMSO) leads to the formation of the corresponding carboxylic acids. The reaction is first order in BIFC. The Michaelis–Menten type kinetics was observed with respect to the aldehyde. The reaction is catalysed by hydrogen ions. The hydrogen-ion dependence has the form: k_obs = a + b[H⁺]. The oxidation of deuterated acetaldehyde, CH₃CDO, exhibited a substantial primary kinetic isotope effect (k_H/k_D = 6.05 at 303 K). Oxidation of acetaldehyde has been studied in eighteen different organic solvents. Various thermodynamic parameters are discussed along with isokinetic temperature. The solvent effect has been analysed using multi parametric equations. A mechanism involving transfer of hydride ion has been suggested. The linear isokinetic correlation implies that all the aldehydes are oxidized by the same mechanism.

Keywords

Aliphatic aldehyde

Benzimidazolium fluorochromate

Kinetics

Oxidation

Solvent effect

Show Related Articles from PubMed

1 Introduction

Chromium compounds have been used in aqueous and non-aqueous medium for the oxidation of a variety of organic compounds. Chromium compounds especially Cr(VI) reagents have been versatile reagents and capable of oxidizing almost all the oxidizable organic functional groups (Wiberg, 1965). The development of newer chromium (VI) reagents for the oxidation of organic substrates continues to be of interest.

A number of new chromium(VI) containing compounds like pyridinium chlorochromate (Corey and Boger, 1978), quinolinium fluorochromate (Dave et al., 2002), quinolinium bromochromate (Nalawaya et al., 2002), quinolinium dichromate (Medien, 2003), pyridinium fluorochromate (Kavitha et al., 2005), imidazolium fluorochromate (Pandurangan et al., 1999), isoquinolinium bromochromate (Vibhute et al., 2009), triphenylmethylposphonium chlorochromate (Hajipour et al., 2005), tributylammonium chlorochromate (Mansoor and Shafi, 2010a), prolinium chlorochromate (Mamaghani et al., 2002), tripropylammonium fluorochromate (Mansoor and Shafi, 2010b, Mansoor, 2011), tetraethyl ammonium bromochromate (Mansoor and Shafi, 2011a), triethylammonium chlorochromate (Mansoor and Shafi, 2014) and tetraethylammonium chlorochromate (Mansoor and Shafi, 2015) have been used to study the kinetics and mechanism of various organic compounds.

The kinetics of oxidation of aliphatic aldehydes have been studied by many reagents such as bis (2,2′-bipyridyl) copper(II) permanganate (Kothari et al., 1992), pyridinium bromochromate (Khanchandani et al., 1996), benzyltrimethyl-ammonium chlorobromate (Bohra et al., 1999), quinolinium fluorochromate (Khurana et al., 1999), 2,2′-bipyridinium chlorochromate (Kumbhat et al., 2000), tetrabutyl ammonium tribromide (Baghmar and Sharma, 2001), morpholinium chlorochromate (Kumbhat et al., 2008) and benzyltriethyl ammonium chlorochromate (Chouhan et al., 2006).

This article focuses on the study of kinetics and mechanism of oxidation of some aliphatic aldehydes by BIFC in nonaqueous media. We have been interested in the kinetic and mechanistic studies of Cr(VI) species. Literature survey reveals that no report is available on the kinetics of oxidation of aliphatic aldehydes by BIFC. Therefore, we studied the kinetics of oxidation of aliphatic aldehydes by BIFC in DMSO. The major objective of this investigation is to study the solvent effect for the substrates undergoing oxidation and to propose a suitable mechanism for the oxidation.

2 Materials and methods

2.1

2.1 Reagents

Benzimidazole and chromium trioxide were obtained from Fluka (Buchs, Switzerland). The aliphatic aldehydes used were formaldehyde, acetaldehyde, propionaldehyde butyraldehyde, monochloro acetaldehyde, dichloro acetaldehyde and trichloro acetaldehyde. The solvents acetonotrile (MeCN), chlorobenzne (CB), chloroform (CF), 1,2-dichloroethane (DCE), dichloromethane (DCM), dimethyl sulfoxide (DMSO), acetone (Me₂CO), dimethylformamide (DMF), butanone (Bu), toluene (TE), cyclohexane (CH), benzene (Bz), nitrobenzene (NB), ethyl acetate (EA), 1,2-dimethoxyethane (DME), tetrahydrofuran (THF),tert-butanol (t-BuOH) and 1,4-dioxane (DO) are of analytical grade and purified by conventional methods (Perrin et al., 1966). Due to non-aqueous nature of the solvent, p-toluene sulfonic acid (TsOH) was used as a source of hydrogen ions. TsOH is a strong acid and in a polar medium like DMSO it is likely to be completely ionized.

2.2

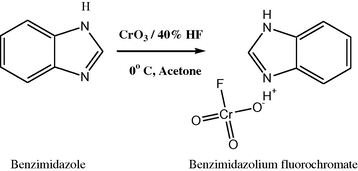
2.2 Preparation of benzimidazolium fluorochromate

Benzimidazolium fluorochromate has been prepared from benzimidazole, 40% hydrofluoric acid and chromium trioxide in the molar ratio 1:1.3:1 at 0 °C. BIFF is obtained as yellow orange crystals. It is non-hygroscopic; light insensitive on storage (Sivamurugan et al., 2005). The purity of BIFC was checked by the iodometric method.

(1)

2.3

2.3 Product analysis

The product analysis was carried out under kinetic conditions. In a typical experiment, acetaldehyde (0.1 mol) and BIFC (0.01 mol) were made up to 100 ml in DMSO in the presence of 0.5 mol dm^–3 TsOH. It was then kept in the dark for 24 h to ensure completion of the reaction. The organic layer was extracted, washed with water, and dried over anhydrous MgSO₄. Ether was removed by warming, and the product is obtained. The boiling point of acetic acid is in agreement with the literature value i.e. 118 °C. The yield of the product is 85%. Iodometric determinations of the oxidation state of chromium in completely reduced reaction mixtures indicated that the oxidation state of the reduced chromium species was 3.94 ± 0.16.

3 Experimental procedures

3.1

3.1 Kinetic measurements

The reactions were followed under pseudo-first-order conditions by keeping a large excess (×15 or greater) of the aldehyde over BIFC. The temperature was kept constant to ±0.1 K. The solvent was DMSO, unless specified otherwise. The reactions were followed by monitoring the decrease in the concentration of BIFC spectrophotometrically using Shimadzu UV–VIS spectrophotometer UV-1800 model at 364 nm for 80% of the reaction. The rate constants, k_obs, were evaluated from the linear (r = 0.990–0.999) plots of log [BIFC] against time. Duplicate kinetic runs showed that the rate constants were reproducible to within ±3%. Multivariate and simple linear regression analyses were carried out by the least-squares method.

4 Results and discussion

The rate and other experimental data were obtained for all the aliphatic aldehydes. The results are summarized here.

4.1

4.1 Order of reaction

The rate of oxidation was found to be first order in [BIFC] and Michaelis–Menten type kinetics was observed with respect to the aldehydes. Linear plots of log k₁ vs. log [RCHO] (Fig. 1) with the slopes (HCHO: slope = 0.893 ± 0.06, r = 0.996; CH₃CHO: slope = 0.926 ± 0.08, r = 0.996; C₂H₅CHO: slope = 0.9120 ± 0.07, r = 0.996; C₃H₇CHO: slope = 0.909 ± 0.05, r = 0.998; CH₂ClCHO: slope = 0.926 ± 0.03, r = 0.998: CHCl₂CHO: slope = 0.923 ± 0.14, r = 0.998 and CCl₃CHO: slope = 0.922 ± 0.09, r = 0.998) demonstrate Michaelis–Menten type kinetics on [RCHO]. The k₁ values at different [RCHO] are given in Table 1. The k₁ values obtained at different concentrations of BIFC reveal that the rates are almost independent of the initial concentration of BIFC. This ensures that the order of the reaction with respect to BIFC is one (see Fig. 2).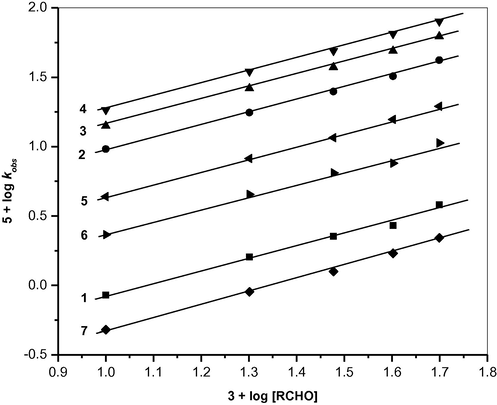

Order plot for the oxidation of aliphatic aldehydes by benzimidazolium fluorochromate in DMSO at 303 K. (1) HCHO; (2) CH3CHO; (3) C2H5CHO; (4) C3H7CHO; (5) CH2ClCHO; (6) CHCl2CHO; (7) CCl3CHO.

Table 1 Effect of variation of [RCHO] and [BIFC] on the rate of reaction at 303 K.

10³[BIFC] (mol dm⁻³)	10²[RCHO] (mol dm⁻³)	10⁵ k₁ (s⁻¹)
10³[BIFC] (mol dm⁻³)	10²[RCHO] (mol dm⁻³)	R = H	CH₃	C₂H₅	C₃H₇	CH₂Cl	CHCl₂	CCl₃
1.0	3.0	2.26	24.90	37.30	49.00	11.60	6.46	1.26
2.0	3.0	2.22	24.94	37.38	49.08	11.52	6.40	1.20
3.0	3.0	2.18	24.98	37.20	49.04	11.50	6.38	1.22
4.0	3.0	2.12	24.86	37.36	49.02	11.58	6.44	1.24
5.0	3.0	2.20	24.80	37.40	49.10	11.50	6.42	1.28
1.0	1.0	0.85	9.60	14.10	18.40	4.36	2.32	0.48
1.0	2.0	1.60	17.60	26.20	34.80	8.22	4.52	0.90
1.0	4.0	2.70	32.10	48.80	65.00	15.70	8.00	1.70
1.0	5.0	3.80	41.86	62.20	80.02	19.50	10.62	2.20
1.0	3.0	2.16	24.78	37.18	48.82	11.48	6.40	1.16^a

[H⁺] = 0.1 mol dm⁻³.

a Contained 0.001 mol dm⁻³ acrylonitrile.

A plot of 1/kobs vs. 1/[RCHO] for the oxidation of aliphatic aldehydes by benzimidazolium fluorochromate in DMSO at 303 K. (1) HCHO; (2) CH3CHO; (3) C2H5CHO; (4) C3H7CHO; (5) CH2ClCHO; (6) CHCl2CHO; (7) CCl3CHO.

4.2

4.2 Stoichiometric studies

The oxidation of aldehydes results in the formation of corresponding carboxylic acids. The overall reaction may be represented as Eq. (2).

(2)

RCHO + {CrO}_{2} {FO}^{-} {BIH}^{+} \to RCOOH + {CrOFO}^{-} {BIH}^{+}

Thus BIFC undergoes a two-electron change.

4.3

4.3 Rate law

The reaction is first order with respect to BIFC. Michaelis–Menten type kinetics were observed with respect to aldehydes (Table 1). A plot of 1/k_obs vs. 1/[aldehyde] is linear with an intercept on the rate ordinate. This indicates the following Eqs. (3) and (4) to represent the mechanism. Eq. (5) represents the rate law.

(3)

Aldehyde + BIFC \overset{K}{⇌} [Complex]

(4)

Complex \overset{k_{2}}{\to} Product

(5)

Rate = k_{2} K [Aldehyde] [BIFC] / (1 + K [Aldehyde])

4.4

4.4 Induced polymerization of acrylonitrile

The oxidation of aldehydes, in an atmosphere of nitrogen, failed to induce polymerization of acrylonitrile. Further, the addition of acrylonitrile did not affect the rate. This indicates that a one-electron oxidation, giving rise to free radicals, is unlikely in the present reaction (Table 1).

4.5

4.5 Effect of acidity

The reaction is catalysed by hydrogen ions. The hydrogen-ion dependence has the following form: Eq. (6). The results are recorded in Table 2.

(6)

k_{obs} = a + b [H^{+}]

The values of a and b for various aldehydes are also presented in Table 2. BIFC may become protonated in the presence of acid. The protonated BIFC may function as an effective oxidant.

(7)

{CrO}_{2} {FO}^{-} {BIH}^{+} + H^{+} ⇌ (OH) {OCrFO}^{-} {BIH}^{+}

The formation of a protonated Cr(VI) species has earlier been postulated in the reactions of structurally similar PCC (Sharma et al., 1997) and PFC (Sharma et al., 1996).

Table 2 Effect of variation of [H⁺] on the rate of reaction at 303 K.

[H⁺] (mol dm⁻³)	R = H	10⁵ k₁ (s⁻¹)
[H⁺] (mol dm⁻³)	R = H	CH₃	C₂H₅	C₃H₇	CH₂Cl	CHCl₂	CCl₃
0.1	2.26	24.90	37.30	49.00	11.60	6.46	1.26
0.2	4.00	40.62	64.02	83.40	19.50	11.08	2.40
0.4	4.80	51.02	77.80	100.80	22.80	14.32	2.80
0.6	4.22	65.00	98.08	118.10	28.50	17.86	3.72
0.8	7.64	79.08	122.00	140.20	35.38	21.80	4.60
1.0	9.20	95.04	148.30	166.60	42.60	26.20	5.58
a	2.4 ± 0.2	25.0 ± 1.1	37.8 ± 2.8	54.1 ± 3.5	11.8 ± 0.4	6.7 ± 0.3	1.3 ± 0.1^m
b	6.7 ± 0.3	18.6 ± 1.9	107.0 ± 4.7	110.9 ± 5.8	29.7 ± 1.5	19.2 ± 0.4	4.2 ± 0.2ⁿ

10²[RCHO] = 3.0 mol dm⁻³; 10³ [BIFC] = 1.0 mol dm⁻³.

m Value of a for the equation: k_obs = a + b[H⁺].

n Value of b for the equation: k_obs = a + b[H⁺].

4.6

4.6 Kinetic isotope effect

The oxidation of deuterated acetaldehyde (CH₃CDO) indicated the presence of a substantial primary kinetic isotope effect. (k_H/k_D = 6.05 at 303 K) (Table 3). This conformed the cleavage of the aldehydic C–H bond in the rate-determining step.

Table 3 Activation parameters and second order rate constants for the oxidation of aliphatic aldehydes by BIFC in DMSO.

Substrates	10⁴ × k₂, s⁻¹				E_a kJ mol⁻¹	−ΔS^# kJ mol⁻¹	ΔH^# Jk mol⁻¹	ΔG^# kJ mol⁻¹(at 303 K)
Substrates	298 K	303 K	308 K	313 K
HCHO	4.9	7.5	10.8	16.3	61.5	110.3 ± 4.2	58.9 ± 1.2	82.4 ± 2.4
CH₃CHO	60.7	83.0	113.6	157.2	48.5	135.2 ± 3.2	46.5 ± 1.0	87.8 ± 1.9
C₂H₅CHO	84.6	124.3	172.7	245.3	54.6	104.3 ± 3.0	53.8 ± 1.0	85.4 ± 1.9
C₃H₇CHO	128.0	163.3	211.3	265.3	37.9	162.5 ± 1.2	35.4 ± 0.4	84.7 ± 0.7
CH₂ClCHO	24.2	38.7	59.0	92.9	71.2	71.59 ± 3.0	66.6 ± 1.0	88.1 ± 1.5
CHCl₂CHO	16.2	21.5	28.4	37.8	43.6	160.3 ± 1.8	41.16 ± 0.6	89.7 ± 1.5
CCl₃CHO	2.7	4.2	6.2	9.8	66.1	100.5 ± 2.6	63.4 ± 0.8	93.8 ± 1.6
CH₃CDO	9.8	13.7	19.7	26.3	52.8	136.0 ± 2.8	49.0 ± 0.9	90.8 ± 1.7
k_H/k_D	6.19	6.05	5.76	5.98

10² [RCHO] = 3.0 mol dm⁻³; 10³ [BIFC] = 1.0 mol dm⁻³; [H⁺] = 0.1 mol dm⁻³.

4.7

4.7 Thermodynamic parameters

The kinetics of oxidation of various aldehydes was studied at four different temperatures viz., 298, 303, 308 and 313 K in DMSO. The second order rate constants were calculated (Table 3). An examination of these data indicates that the oxidation is accelerated by electron releasing substituents and retarded by electron withdrawing substituents in the carbon chain. The order of the reactivity is as follows:

HCHO < CH₃CHO < C₂H₅CHO < C₃H₇CHO; and
CH₃CHO > CH₂ClCHO > CHCl₂CHO > CCl₃CHO.

The Arrhenius plot of log k₂ vs. 1/T is found to be linear. The energy of activation values for all the aldehydes were calculated and presented in Table 3. Statistical analysis of the Eyring equation clearly confirms that the standard errors of ΔH^# and ΔS^# correlate (Lente et al., 2005). The entropy of activation is negative for all the aldehydes.

4.8

4.8 Isokinetic relationship

There is no significant isokinetic relationship between the activation enthalpies and entropies of the oxidation of seven aldehydes (r² = 0.6642). A correlation between the calculated values of enthalpies and entropies of activation is often vitiated by the experimental errors associated with them. Exner, (1964a,b) has suggested an alternative method of testing the validity of isokinetic relationship.

The reaction is neither isoenthalpic nor isoentropic but complies with the compensation law also known as the isokinetic relationship.

(8)

Δ H^{#} = Δ H^{o} + β Δ S^{#}

The isokinetic temperature β is the temperature at which all the compounds of the series react equally fast. The isokinetic relationship is tested by plotting the logarithms of rate constants at two different temperatures (T₂ > T₁) against each other according to Eq. (9).

(9)

\log k (at T_{2}) = a + b \log k (at T_{1})

The linear relationship in Exner plots (Exner, 1964a,b, 1973 ) at 4 + log k₂ (303 K) and 4 + log k₂ (313 K) observed in the present study implies the validity of the isokinetic relationship. Exner’s plot is shown in Fig. 3 (slope = 0.930, r = 0.996, isokinetic temperature = 558 K). The linear isokinetic correlation implies that all the aldehydes are oxidized by the same mechanism and are governed by the changes in both the enthalpy and entropy of the activation (Leffler and Grunwald, 1963).

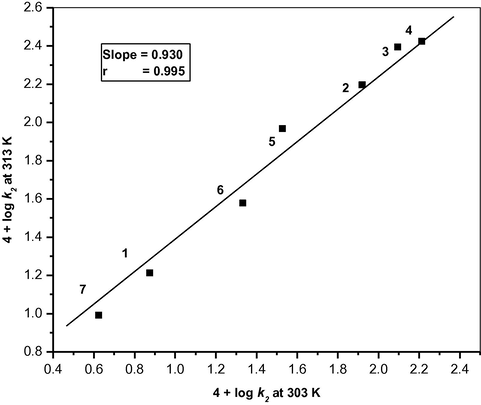
Exner’s plot for the oxidation of aliphatic aldehydes by benzimidazolium fluorochromate in DMSO between 4 + log k2 (at 313 K) and 4 + log k2 (at 303 K). (1) HCHO; (2) CH3CHO; (3) C2H5CHO; (4) C3H7CHO; (5) CH2ClCHO; (6) CHCl2CHO; (7) CCl3CHO.

4.9

4.9 Solvent – reactivity correlation

The rate of oxidation of aliphatic aldehydes was determined in 18 different organic solvents. The choice of the solvents was limited by the solubility of BIFC and its reactivity with aliphatic aldehydes. There was no noticeable reaction with the solvents chosen. The kinetics were similar in all the solvents. The values of k₂ at four different temperatures were determined. The thermodynamic parameters were also calculated for the oxidation of acetaldehyde in 18 different organic solvents and the values are recorded in Table 4. Negative entropy of activation indicates a greater degree of ordering in the transition state than in the initial state, due to an increase in solvation during the activation process. The isokinetic relationship of acetaldehyde in 18 different organic solvent is shown in Fig. 4. The existence of a linear relationship (slope = 0.942, r = 0.997, isokinetic temperature = 675 K) between 4 + log k₂ (303 K) and 4 + log k₂ (313 K) indicates that a common mechanism is operating in all studied solvent systems.

Table 4 Activation parameters and second order rate constants for the oxidation of acetaldehyde by BIFC in eighteen different solvents.

Solvents	10⁴k₂ (dm³ mol⁻¹ s⁻¹)				E_a kJ mol⁻¹	−ΔS^# kJ mol⁻¹	ΔH^# Jk mol⁻¹	ΔG^# kJ mol⁻¹(at 303 K)
Solvents	298 K	303 K	308 K	313 K
MeCN	72.02	98.00	128.40	166.80	43.3	149.3 ± 2.0	40.8 ± 0.6	86.0 ± 1.2
CB	12.85	18.20	25.20	34.08	50.4	139.6 ± 1.3	47.9 ± 0.4	90.2 ± 0.8
CF	15.08	22.60	33.00	49.50	61.1	102.4 ± 3.1	58.6 ± 1.0	89.6 ± 1.9
DCE	14.90	21.70	31.30	43.80	55.9	121.0 ± 1.3	53.0 ± 0.4	89.7 ± 0.8
DCM	17.80	23.30	40.50	59.90	62.6	96.1 ± 1.4	60.1 ± 0.4	89.2 ± 0.8
DMSO	60.70	83.00	113.60	157.20	48.5	135.1 ± 3.0	46.5 ± 1.0	87.7 ± 1.9
Me₂CO	15.80	25.40	39.40	63.00	71.1	68.5 ± 3.8	68.5 ± 1.2	89.3 ± 2.3
DMF	33.60	43.20	56.00	72.60	39.8	167.3 ± 1.9	37.3 ± 0.6	88.0 ± 1.2
Bu	13.20	18.60	24.90	35.50	50.6	139.4 ± 4.0	47.9 ± 1.4	91.0 ± 2.6
TE	4.90	7.40	10.50	15.00	57.5	123.7 ± 2.8	55.5 ± 0.9	93.0 ± 1.7
CH	0.54	0.86	1.40	2.00	69.1	103.0 ± 3.7	66.6 ± 1.3	97.8 ± 2.4
Bz	5.70	8.20	11.00	15.50	51.1	143.6 ± 4.1	48.6 ± 1.4	92.1 ± 2.6
NB	25.50	34.20	45.10	60.50	44.4	154.1 ± 2.3	41.7 ± 0.8	88.4 ± 1.5
EA	5.94	8.80	12.32	17.74	56.2	126.9 ± 2.9	53.6 ± 0.9	92.1 ± 1.8
DME	4.00	6.20	9.18	13.70	63.2	105.8 ± 1.9	60.9 ± 0.6	92.9 ± 1.2
THF	14.42	19.60	27.44	37.30	49.8	142.2 ± 2.7	46.7 ± 0.9	89.8 ± 1.7
t-BuOH	6.62	10.10	15.02	21.60	61.3	108.9 ± 1.9	58.6 ± 0.6	91.6 ± 1.2
DO	8.80	14.00	21.80	33.20	68.6	68.3 ± 1.6	70.1 ± 0.6	90.8 ± 1.1

10² [RCHO] = 3.0 mol dm⁻³; 10³ [BIFC] = 1.0 mol dm⁻³; [H⁺] = 0.1 mol dm⁻³.

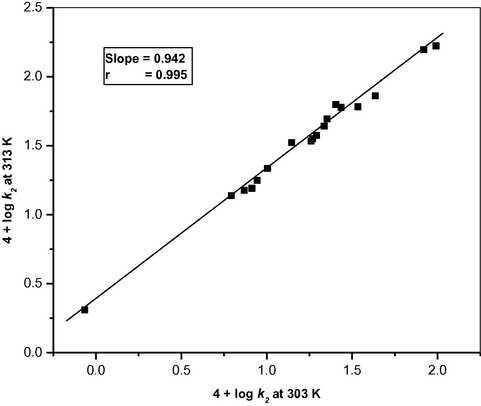
Exner’s plot for the oxidation of acetaldehyde by benzimidazolium fluorochromate in eighteen different solvents between 4 + log k2 (at 313 K) and 4 + log k2 (at 303 K).

The influence of solvent on the rate of any reaction can be described in terms of solvation which is a stabilization process. Two viewpoints have been established on the solvation phenomenon. According to the first, a solvent is considered as a homogeneous continuum which surrounds the solute molecules and exerts long range interactions. The strength of these interactions with the solute molecules is described in terms of macroscopic physical properties of the solvent like dielectric constant (ε) and refractive index (η). Using idealized theories, the solvent relative permittivity is often predicted to serve as a quantitative measure of solvent polarity. However, this approach is often inadequate since these theories regard solvents as a non-structured continuum, not composed of individual solvent molecules with their own solvent interactions and they do not take into account specific solute–solvent interactions such as hydrogen bonding and electron pair donor/electron pair acceptor interactions, which often play a dominating role in solute–solvent interactions (Bhuvaneswari and Elango, 2006).

No single microscopic physical parameter could possibly account for the multitude of solute–solvent interactions on the molecular microscopic level. Thus, bulk solvent properties like the relative permittivity and ionizing power will poorly describe the microenvironment around the reacting species, which governs the stability of transition state and hence the rate of the reaction. Hence, there have been a variety of attempts to quantify different aspects of solvent polarity and then use the resultant parameters to interpret solvent effects on reactivity through multiple regression. Various attempts for the above solvent–solvent–solute interactions based on linear salvation energy relationship (LSER) have been developed (Shorter, 1982).

According to the second view point, a solvent is considered to be anisotropic and inhomogeneous which exerts short range forces on the solute molecules. These forces are chemical in nature, and result in the formation of solvation complexes through donor–acceptor bonds which are localized and directed in space. The strength of these interactions is described in terms of solvation parameters namely hydrogen bond donor acidity (α), hydrogen bond acceptor basicity (β), etc., Thus the solvent can solvate the solute by exhibiting any of these interactions with the specific sites in the solute. Hence, the effect of solvent on the rate of the reaction can be described in terms of multi parametric equation which involves different solvation parameters given by Kamlet et al. (1983).

4.9.1

4.9.1 The Kamlet–Taft method for the examination of solvent effect

In order to obtain a deeper insight into the various solvent–solvent–solute interactions, which influence reactivity, the solvatochromic comparison method developed by Kamlet et al. (1983) has been used. This method may be used to unravel, quantify, correlate and rationalize multiple interacting solvent effects on reactivity. The kinetic data were correlated with the solvatochromic parameters α, β and π^∗ characteristic of different solvents in the form of following LSER:

(10)

\log k_{2} = A_{0} + p π^{*} + b β + a α

where π^∗ is an index of solvent dipolarity/polarizability, which measures the ability of the solvent to stabilize a charge or a dipole by virtue of its dielectric effect, α is the solvent HBD (hydrogen bond donor) acidity, β is the solvent HBA (hydrogen bond acceptor) basicity of the solvent in a solute to solvent hydrogen bond and A₀ is the intercept term.

The rate constants of oxidation, k₂, in 18 solvents were correlated in terms of the linear salvation energy relationship Eq. (10) of Kamlet et al. (1983).

Kamlet et al. (1981) established that the effect of a solvent on the reaction rate should be given in terms of the following properties: (i) the behaviour of the solvent as a dielectric, facilitating the separation of opposite charges in the transition state, (ii) the ability of the solvent to donate a proton in a solvent-to-solute hydrogen bond and thus stabilize the anion in transition state and (iii) the ability of the solvent to donate an electron pair and therefore stabilize the initial aliphatic aldehyde, by way of a hydrogen bond between the aldehydic proton and the solvent electron pair. The parameter π^∗ is an appropriate measure of the first property, while the second and third properties are governed by the parameters α and β respectively. The solvent parameters (π^∗, α and β) are taken from the literature (Kamlet et al., 1983) and are given in Table 5. The linear dependence (LSER) on the solvent properties was used to correlate and predict a wide variety of solvent effect.

Table 5 Solvent parameters for the eighteen different solvents (Kamlet et al., 1983).

Solvents	π^∗	α	β
Acetonitrile	0.75	0.19	0.31
Chlorobenzene	0.71	0.00	0.07
Chloroform	0.58	0.44	0.00
1,2-Dichloroethane	0.81	0.00	0.00
Dichloromethane	0.82	0.30	0.00
DMSO	1.00	0.00	0.76
Acetone	0.71	0.08	0.48
DMF	0.88	0.00	0.69
Butanone	0.67	0.06	0.48
Toluene	0.54	0.00	0.11
Cyclohexane	0.00	0.00	0.00
Benzene	0.59	0.00	0.10
Nitrobenzene	1.01	0.00	0.39
Ethyl Acetate	0.55	0.00	0.45
1,2-Dimethoxyethane	0.53	0.00	0.41
THF	0.58	0.00	0.55
tert-Butyl Alcohol	0.41	0.68	1.01
1,4-Dioxane	0.55	0.00	0.37

In order to explain the kinetic results through the solvent polarity and basicity or acidity, the rate constants were correlated with the solvatochromic parameters π^∗, α and β using total solvatochromic equation, Eq. (10). The correlation of kinetic data was realized by means of multiple linear regression analysis. It was found that the rate constants in 18 solvents showed moderate correlation with the π^∗, α and β solvent parameters. The results of correlation analysis in terms of Eq. (10), a biparametric equation involving π^∗ and β are given below in Eqs. (11)–(15).

(11)

\begin{matrix} \log k_{2} = (- 4.05 \pm 0.16) + (1.80 \pm 0.22) π^{*} + (0.14 \pm 0.11) β + (0.49 \pm 0.27) α \\ R^{2} = 0.83; SD = 0.11; n = 18; ψ = 0.28 \end{matrix}

(12)

\begin{matrix} \log k_{2} = (- 3.98 \pm 0.12) + (1.75 \pm 0.20) π^{*} + (0.22 \pm 0.16) β \\ R^{2} = 0.80; SD = 0.12; n = 18; ψ = 0.18 \end{matrix}

(13)

\begin{matrix} \log k_{2} = (- 4.03 \pm 0.16) + (1.84 \pm 0.22) π^{*} + (0.55 \pm 0.22) β + (0.55 \pm 0.22) α \\ R^{2} = 0.81; SD = 0.16; n = 18; ψ = 0.12 \end{matrix}

(14)

\begin{matrix} \log k_{2} = (- 3.89 \pm 0.22) + (1.62 \pm 0.12) π^{*} \\ r^{2} = 0.83; SD = 0.14; n = 18; ψ = 0.20 \end{matrix}

(15)

\begin{matrix} \log k_{2} = (- 3.41 \pm 0.16) + (0.52 \pm 0.28) β \\ r^{2} = 0.77; SD = 0.26; n = 18; ψ = 0.78 \end{matrix}

Here n is the number of data points and ψ is the Exner’s statistical parameter (Exner, 1966).

Kamlet et al. (1983) triparametric equation explains ca. 83% of the effect of solvent on the oxidation. However, by Exner’s criterion Exner (1966) the correlation is not even satisfactory (cf. Eq. (15)). The major contribution is of solvent polarity. It alone accounted for ca. 77% of the data. Both α and β play relatively minor roles.

4.9.2

4.9.2 The Swain’s method for the examination of solvent effect

The data on solvent effect were analysed in terms of Swain’s equation Swain et al. (1983) of cation-and anion-solvating concept of the solvents also.

(16)

\log k_{2} = aA + bB + C

Here A represents the anion-solvating power of the solvent and B the cation-solvating power. C is the intercept term. (A + B) is postulated to represent the solvent polarity. The rates in different solvents were analysed in terms of Eq. (16), separately with A and B and with (A + B).

(17)

\begin{matrix} \log k_{2} = (0.58 \pm 0.01) A + (1.72 \pm 0.04) B - 3.88 \\ R^{2} = 0.996; SD = 0.08; n = 18; ψ = 0.18 \end{matrix}

(18)

\begin{matrix} \log k_{2} = 0.36 (\pm 0.27) A - 2.88 \\ r^{2} = 0.0305; SD = 0.06; n = 18; ψ = 0.58 \end{matrix}

(19)

\begin{matrix} \log k_{2} = 1.66 (\pm 0.16) B - 3.90 \\ r^{2} = 0.952; SD = 0.22; n = 18; ψ = 0.25 \end{matrix}

(20)

\begin{matrix} \log k_{2} = (1.36 \pm 0.12) (A + B) - 4.22 \\ r^{2} = 0.8816; SD = 0.18; n = 18; ψ = 0.60 \end{matrix}

The rates of oxidation of acetaldehyde in different solvents showed an excellent correlation in Swain’s equation with the cation-solvating power playing the major role. In fact, the cation solvation alone accounts for ca. 95% of the data. The correlation with anion- solvating power was very poor. The solvent polarity, represented by (A + B), also accounted for ca. 88% of the data.

4.10

4.10 Mechanism of oxidation

A one-electron oxidation, giving rise to free radicals, is unlikely in view of the failure to induce polymerization of acrylonitrile. Removal of the hydrogen was confirmed by the kinetic isotope effect observed for the oxidation of acetaldehyde (k_H/k_D = 6.05). Therefore, the removal of hydrogen as hydride-ion resulting in an electron-deficient species in the rate-determining step, is indicated. The hydride ion transfer may take place either by a cyclic process via an ester intermediate or by an acyclic one-step bimolecular process. The hydride-transfer mechanism is also supported by the major role of cation-solvating power of the solvents.

An analysis of the temperature dependence of the kinetic isotope effect by the method of Kwart and Nickel (1973) showed that the loss of hydrogen proceeds through a concerted cyclic process. The data for protio and deuterio-acetaldehyde were fitted to the familiar expression $k_{H} / k_{D} = A_{H} / A_{D} \exp (- Δ Ea / RT)$ or,

(21)

\log (k_{H} / k_{D}) = \log (A_{H} / A_{D}) - Δ Ea / RT

The results showed that the activation energy difference for k_H/k_D is 4.3 kJ which agrees well with the zero-point energy difference for the respective C–H and C–D bonds (4.3 kJ mol^–1) and the entropy of activation of the respective reactions is equal. This directly corresponds to the properties of a symmetrical transition state (Kwart and Latimer, 1971; Kwart and Slutsky, 1972). The similar phenomena were observed earlier in the oxidation of diols by BPCC (Loonker et al., 1997) and that of alcohols by PFC (Banerji, 1988). However, the observed Michaelis–Menten type kinetics with respect to the aldehyde leads to suggest the formation of 1:1 complex by a nucleophilic attack of aldehydic oxygen on chromium in a rapid pre-equilibrium. The nature of the complex suggested is similar to that reported in the oxidation of aromatic aldehydes by PFC (Agarwal et al., 1991). The hydrogen-ion dependence has the form k_obs = a + b[H⁺]. The observed acid-dependence of the reaction points to a rapid reversible protonation of the intermediate complex prior to its disproportionation. A mechanism depicted in Scheme 1 accounts for the experimental results.

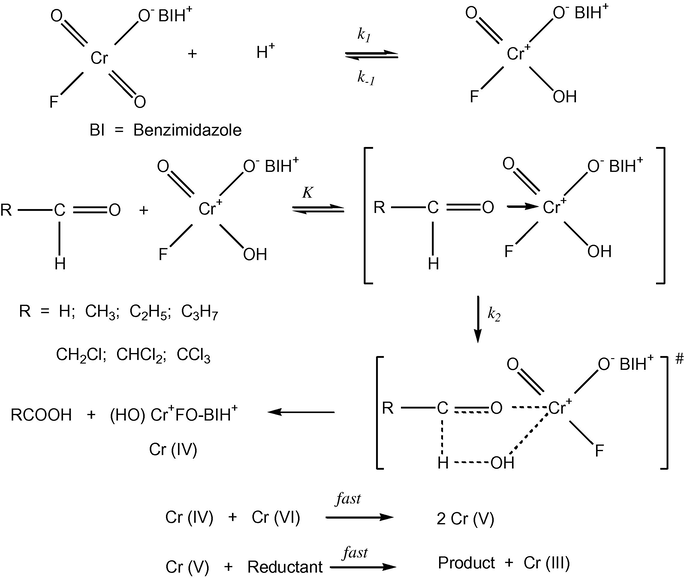
Mechanism for the oxidation of aliphatic aldehydes by benzimidazolium fluorochromate.

The order of reactivity for the oxidation of aliphatic aldehydes by BIFC observed was: HCHO < CH₃CHO < C₂H₅CHO < C₃H₇CHO, showing that the rate of oxidation was dependent on the length of the alkyl chain of the aldehydes. Furthermore, electron-releasing groups (R = H, CH₃, C₂H₅, and C₃H₇,) accelerated the oxidation process by increasing the electron availability at the oxygen of the aldehydic carbonyl group. This facilitated the attack of the electrophile i.e. protonated BIFC.

A cyclic structure for the reaction intermediate would explain all the features of the oxidation reaction. If the chromium was coordinated through the oxygen atom of the aldehyde, then the formation of the chromate ester would be facilitated. This would increase the ease of oxidation, and the conversion to the corresponding carboxylic acid could be rationalized. Bordwell (1974) has given cogent evidence against the occurrence of concerted one-step bimolecular process of hydrogen transfer and it is clear that in the present reaction also, the hydrogen transfer does not occur by an acyclic bimolecular process. The only truly symmetrical processes involving linear transfer of hydrogen are intrinsically concerted sigmatropic reactions characterized by transfer with a cyclic transition state (Woodward and Hoffman, 1969). The second step of the reaction was the transfer of two electrons in a cyclic system. This electrocyclic mechanism for the oxidation of aldehyde by BIFC involved six electrons, being a Hückel type system, is an allowed process (Litller, 1971). Therefore, it can be concluded that in the oxidation of aldehyde by BIFC, the hydride-ion transfer occurs via a cyclic transition state.

The manner of electron transfer has to be established. The first step involved the nucleophilic attack of aldehydic-oxygen electrons on electron-deficient chromium atom to form an intermediate complex. This complex then undergoes unimolecular decomposition in the slow step. The transition state involves the bonding of hydrogen atom to both the aldehydic-carbon and the OH group attached to chromium. The electron flow in a cyclic transition state has been considered assuming that the hydrogen atom is removed as hydride-ion. Thus, the process of electron transfer takes place through the carbon–hydrogen–oxygen–chromium bond. This would facilitate the formation of a carbocationic species by reverting back the nucleophilic attack of aldehydic oxygen. The similar type of mechanism is reported for the oxidation of aldehydes by BIDC (Kumar et al., 2011).

The proposed mechanism is, however, supported by the observed negative entropy of activation. As the charge separation takes place in the transition state, the two ends become highly solvated. This results in an immobilization of a large number of solvent molecules, reflected in the loss of entropy. The negative entropy of activation additionally accounts for the influence of solvent. In this mechanism Cr(VI) undergoes two electron reduction to form Cr(IV). This Cr(IV) is likely to react with another Cr(VI) to generate Cr(V). The Cr(V) is then reduced in a fast step to produce Cr(III). Such a sequence of reactions in Cr(VI) oxidations is well known [1] (Scheme 1).

5 Conclusion

The oxidation of seven aliphatic aldehydes by benzimidazolium fluorochromate (BIFC) in DMSO was studied. The oxidation leads to the formation of the corresponding carboxylic acids. The reaction is first order with respect to BIFC. The reaction exhibited Michaelis–Menten type kinetics with respect to the aldehydes. The reaction is catalysed by hydrogen ions. The hydrogen-ion dependence has the form: k_obs = a + b[H⁺]. An examination of rate data indicates that the oxidation is accelerated by electron releasing substituents and retarded by electron withdrawing substituents in the carbon chain. Oxidation of acetaldehyde was studied in 18 different organic solvents. The solvent effect has been analysed using Kamlet’s and Swain’s multi-parametric equation. Removal of the hydrogen was confirmed by the kinetic isotope effect observed for the oxidation of acetaldehyde. The linear isokinetic correlation implies that all the aldehydes are oxidized by the same mechanism.

Acknowledgements

The author Mansoor is thankful to Dr. P.K. Sharma, Professor, Jai Narain Vyas University, Jodhpur – India for his valuable suggestions. The authors also wish to thank to C. Abdul Hakeem College Management, Melvisharam-India, for the facilities and support from the DST-FIST (Government of India) sponsored Department.

References

Agarwal S., Chowdhury K., Banerji K.K., . J. Org. Chem.. 1991;56:5111-5113.
Baghmar M., Sharma P.K., . Int. J. Chem. Kinet.. 2001;33:390.
Banerji K.K., . J. Chem. Soc. Perkin Trans.. 1988;2:547.
Bhuvaneswari D.S., Elango K.P., . Int. J. Chem. Kinet.. 2006;38:166-175.
Bohra A., Sharma P.K., Banerji K.K., . J. Chem. Res.. 1999(S);308(M):1343.
Bordwell F.G., . Acc. Chem. Res.. 1974;5:374.
Chouhan K., Prasad P.R., Sharma P.K., . J. Ind. Chem. Soc.. 2006;83(2):191-194.
Corey E.J., Boger D.L., . Tet. Lett.. 1978;28:2461.
Dave I., Sharma V., Banerji K.K., . J. Indian Chem. Soc.. 2002;79:347.
Exner O., . Collect Czech Chem. Commun.. 1964;29:1094.
Exner O., . Nature. 1964:488.
Exner O., . Collect Czech Chem. Commun.. 1966;31:3222.
Exner O., Streitwiser J.R., Talt R.W., . Progress in Physical Organic Chemistry. New York: John Wiley; 1973. p 41
Hajipour A.R., Khazdooz L., Ruoho A.E., . J. Iranian Chem. Soc.. 2005;2:315-318.
Kamlet M.J., Abboud J.L.M., Taft R.W., . Prog. Phys. Org. Chem.. 1981;13:485.
Kamlet M.J., Abboud J.L.M., Abraham M.H., Taft R.W., . J. Org. Chem.. 1983;48:2877. and references cited therein
Kavitha S., Pandurangan A., Alphonse I., . Indian J. Chem.. 2005;44A:715.
Khanchandani R., Sharma P.K., Banerji K.K., . Indian J. Chem.. 1996;35A:576.
Khurana M., Sharma P.K., Banerji K.K., . React. Kinet. Catal. Lett.. 1999;67:341.
Kothari S., Sharma V., Sharma P.K., Banerji K.K., . Proc. Indian Acad. Sci., Chem. Sci.. 1992;104:583.
Kumar P., Panday D., Kothari S., . Croat. Chem. Acta.. 2011;84(1):53-61.
Kumbhat V., Sharma P.K., Banerji K.K., . Indian J. Chem.. 2000;39A:1169-1174.
Kumbhat V., Sharma P.K., Banerji K.K., . J. Ind. Chem. Soc.. 2008;85(8):857-861.
Kwart H., Latimer M.C., . J. Am. Chem. Soc.. 1971;93:3770.
Kwart H., Nickel J.H., . J. Am. Chem. Soc.. 1973;95:3394.
Kwart H., Slutsky J., . J. Chem. Soc., Chem. Commun. 1972:1182.
Leffler J.F., Grunwald E., . Rates and Equilibrium of Organic Reactions. New York: Wiley; 1963.
Lente G., Fabian I., Poe A.J., . New J. Chem.. 2005;29:759-760.
Litller J.S., . Tetrahedron. 1971;27:81.
Loonker K., Sharma P.K., Banerji K.K., . J. Chem. Res.(S). 1997;242(M):1663.
Mamaghani M., Shirini F., Parsa F., . Russ. J. Org. Chem.. 2002;38:1113-1115.
Mansoor S.S., . E.-J. Chem.. 2011;8(2):643-648.
Mansoor S.S., Shafi S.S., . Reac. Kinet. Mech. Cat.. 2010;100:21-30.
Mansoor S.S., Shafi S.S., . J. Mol. Liq.. 2010;155:85-90.
Mansoor S.S., Shafi S.S., . Z. Phys. Chem.. 2011;225:249-260.
Mansoor, S.S., Shafi, S.S., 2014. Oxidation of aliphatic alcohols by triethylammonium chlorochromate in non aqueous medium – A kinetic and mechanistic study. Arab. J. Chem. 7, 312–318.
Mansoor, S.S., Shafi, S.S., 2015. Oxidation of methionine by tetraethylammonium chlorochromate in non-aqueous media – A kinetic and mechanistic study. Arab. J. Chem. 8, 480–486.
Medien H.A.A., . Z. Naturforsch.. 2003;58b:1201.
Nalawaya N., Jain A., Hiran B.L., . J. Indian Chem. Soc.. 2002;79:587.
Pandurangan A., Rajkumar G.A., Arbindoo B., Murugesan V., . Indian J. Chem.. 1999;38B:99.
Perrin D.D., Armarego W.L., Perrin D.R., . Purification of Organic Compounds. Oxford: Pergamon Press; 1966.
Sharma V., Sharma P.K., Banerji K.K., . J. Chem. Res. (S) 1996:290.
Sharma V., Sharma P.K., Banerji K.K., . J. Indian Chem. Soc.. 1997;74:607.
Shorter J., . Correlation analysis of organic reactivity. Letchworth: Research Studies Press; 1982.
Sivamurugan V., Rajkumar G.A., Arabindoo B., Murugesan V., . Indian J. Chem.. 2005;44A:144.
Swain C.G., Swain M.S., Powel A.L., Alunni S., . J. Am. Chem. Soc.. 1983;105:502.
Vibhute A.Y., Patwari S.B., Khansole S.V., Vibhute Y.B., . Chin. Chem. Lett.. 2009;20:256.
Wiberg W.B., . Oxidation in Organic Chemistry. New York: Academic Press; 1965.
Woodward R.W., Hoffman R., . Angew Chem., Int. Ed. Engl.. 1969;8:781.

Show Sections

[1] Agarwal S., Chowdhury K., Banerji K.K., . J. Org. Chem.. 1991;56:5111-5113.

[2] Baghmar M., Sharma P.K., . Int. J. Chem. Kinet.. 2001;33:390.

[3] Banerji K.K., . J. Chem. Soc. Perkin Trans.. 1988;2:547.

[4] Bhuvaneswari D.S., Elango K.P., . Int. J. Chem. Kinet.. 2006;38:166-175.

[5] Bohra A., Sharma P.K., Banerji K.K., . J. Chem. Res.. 1999(S);308(M):1343.

[6] Bordwell F.G., . Acc. Chem. Res.. 1974;5:374.

[7] Chouhan K., Prasad P.R., Sharma P.K., . J. Ind. Chem. Soc.. 2006;83(2):191-194.

[8] Corey E.J., Boger D.L., . Tet. Lett.. 1978;28:2461.

[9] Dave I., Sharma V., Banerji K.K., . J. Indian Chem. Soc.. 2002;79:347.

[10] Exner O., . Collect Czech Chem. Commun.. 1964;29:1094.

[11] Exner O., . Nature. 1964:488.

[12] Exner O., . Collect Czech Chem. Commun.. 1966;31:3222.

[13] Exner O., Streitwiser J.R., Talt R.W., . Progress in Physical Organic Chemistry. New York: John Wiley; 1973. p 41

[14] Hajipour A.R., Khazdooz L., Ruoho A.E., . J. Iranian Chem. Soc.. 2005;2:315-318.

[15] Kamlet M.J., Abboud J.L.M., Taft R.W., . Prog. Phys. Org. Chem.. 1981;13:485.

[16] Kamlet M.J., Abboud J.L.M., Abraham M.H., Taft R.W., . J. Org. Chem.. 1983;48:2877. and references cited therein

[17] Kavitha S., Pandurangan A., Alphonse I., . Indian J. Chem.. 2005;44A:715.

[18] Khanchandani R., Sharma P.K., Banerji K.K., . Indian J. Chem.. 1996;35A:576.

[19] Khurana M., Sharma P.K., Banerji K.K., . React. Kinet. Catal. Lett.. 1999;67:341.

[20] Kothari S., Sharma V., Sharma P.K., Banerji K.K., . Proc. Indian Acad. Sci., Chem. Sci.. 1992;104:583.

[21] Kumar P., Panday D., Kothari S., . Croat. Chem. Acta.. 2011;84(1):53-61.

[22] Kumbhat V., Sharma P.K., Banerji K.K., . Indian J. Chem.. 2000;39A:1169-1174.

[23] Kumbhat V., Sharma P.K., Banerji K.K., . J. Ind. Chem. Soc.. 2008;85(8):857-861.

[24] Kwart H., Latimer M.C., . J. Am. Chem. Soc.. 1971;93:3770.

[25] Kwart H., Nickel J.H., . J. Am. Chem. Soc.. 1973;95:3394.

[26] Kwart H., Slutsky J., . J. Chem. Soc., Chem. Commun. 1972:1182.

[27] Leffler J.F., Grunwald E., . Rates and Equilibrium of Organic Reactions. New York: Wiley; 1963.

[28] Lente G., Fabian I., Poe A.J., . New J. Chem.. 2005;29:759-760.

[29] Litller J.S., . Tetrahedron. 1971;27:81.

[30] Loonker K., Sharma P.K., Banerji K.K., . J. Chem. Res.(S). 1997;242(M):1663.

[31] Mamaghani M., Shirini F., Parsa F., . Russ. J. Org. Chem.. 2002;38:1113-1115.

[32] Mansoor S.S., . E.-J. Chem.. 2011;8(2):643-648.

[33] Mansoor S.S., Shafi S.S., . Reac. Kinet. Mech. Cat.. 2010;100:21-30.

[34] Mansoor S.S., Shafi S.S., . J. Mol. Liq.. 2010;155:85-90.

[35] Mansoor S.S., Shafi S.S., . Z. Phys. Chem.. 2011;225:249-260.

[36] Mansoor, S.S., Shafi, S.S., 2014. Oxidation of aliphatic alcohols by triethylammonium chlorochromate in non aqueous medium – A kinetic and mechanistic study. Arab. J. Chem. 7, 312–318.

[37] Mansoor, S.S., Shafi, S.S., 2015. Oxidation of methionine by tetraethylammonium chlorochromate in non-aqueous media – A kinetic and mechanistic study. Arab. J. Chem. 8, 480–486.

[38] Medien H.A.A., . Z. Naturforsch.. 2003;58b:1201.

[39] Nalawaya N., Jain A., Hiran B.L., . J. Indian Chem. Soc.. 2002;79:587.

[40] Pandurangan A., Rajkumar G.A., Arbindoo B., Murugesan V., . Indian J. Chem.. 1999;38B:99.

[41] Perrin D.D., Armarego W.L., Perrin D.R., . Purification of Organic Compounds. Oxford: Pergamon Press; 1966.

[42] Sharma V., Sharma P.K., Banerji K.K., . J. Chem. Res. (S) 1996:290.

[43] Sharma V., Sharma P.K., Banerji K.K., . J. Indian Chem. Soc.. 1997;74:607.

[44] Shorter J., . Correlation analysis of organic reactivity. Letchworth: Research Studies Press; 1982.

[45] Sivamurugan V., Rajkumar G.A., Arabindoo B., Murugesan V., . Indian J. Chem.. 2005;44A:144.

[46] Swain C.G., Swain M.S., Powel A.L., Alunni S., . J. Am. Chem. Soc.. 1983;105:502.

[47] Vibhute A.Y., Patwari S.B., Khansole S.V., Vibhute Y.B., . Chin. Chem. Lett.. 2009;20:256.

[48] Wiberg W.B., . Oxidation in Organic Chemistry. New York: Academic Press; 1965.

[49] Woodward R.W., Hoffman R., . Angew Chem., Int. Ed. Engl.. 1969;8:781.

Oxidation of aliphatic aldehydes by benzimidazolium fluorochromate in non aqueous medium – A kinetic and mechanistic study

Abstract

Keywords

Aliphatic aldehyde

Benzimidazolium fluorochromate

Kinetics

Oxidation

Solvent effect

1 Introduction

2 Materials and methods

2.1 Reagents

2.2 Preparation of benzimidazolium fluorochromate

2.3 Product analysis

3 Experimental procedures

3.1 Kinetic measurements

4 Results and discussion

4.1 Order of reaction

4.2 Stoichiometric studies

4.3 Rate law

4.4 Induced polymerization of acrylonitrile

4.5 Effect of acidity

4.6 Kinetic isotope effect

4.7 Thermodynamic parameters

4.8 Isokinetic relationship

4.9 Solvent – reactivity correlation

4.9.1 The Kamlet–Taft method for the examination of solvent effect

4.9.2 The Swain’s method for the examination of solvent effect

4.10 Mechanism of oxidation

5 Conclusion

Acknowledgements

References

Suggested read for related articles: